The education field has seen decades of scrutiny, debate, and reform that has centered on the importance of procedural fluency versus conceptual understanding with respect to student learning and proficiency in math. Many educators may see these two sides pitted against each other or framed as mutually exclusive. But what is usually lost in the debate is that procedural fluency and conceptual knowledge are two of the National Research Council’s five strands of math proficiency—they’re both essential.
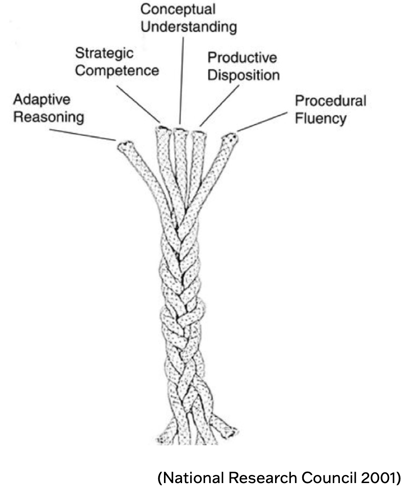
Traditional math curricula often focus on procedural knowledge and fluency. This approach focuses on developing students’ proficiency with facts and algorithms and relies on specific step-by-step methods for solving problems. It also values efficiency and speed as students practice and memorize facts and action sequences until they become automatic.
In recent years, educators have focused more on the need to develop students’ conceptual knowledge and understanding of math. This approach emphasizes that students should understand the ideas, reasoning, and frameworks underlying
the discipline of math. It highlights the need to build connections among different math facts and procedures and aims to help students learn why different math concepts are important and identify the kinds of contexts in which they are useful.
But rather than an either/or proposition, procedural fluency and conceptual knowledge are interwoven and interdependent components of learning math successfully, and numerous research studies shed light on the role they play in student learning.
- Memorization is a valuable tool and frees up working memory for more complex thinking. In fact, neuroscientists tracked a group of young students for a year and used brain-scanning techniques to understand the relationship between different problem-solving approaches and structures in the brain. Over the course of the year, as students progressed from counting on fingers to simply remembering the answers to basic math problems, the scientists saw physical changes in the students’ brains as the hippocampus (a key brain structure for memory) gradually took over from the prefrontal parietal cortex (a key structure for executive processing and reasoning). In other words, “as young math students memorize the basics, their brains reorganize to accommodate the greater demands of more complex math” (Qin et al. 2014).
-
Conceptual knowledge of math allows students to use math more flexibly and better retain what they have learned. In one study, for example, researchers assessed a group of grade 4 and 5 students’ conceptual and procedural knowledge of equivalence before and after a brief lesson. Students either received a lesson on the concept of equivalence and or a lesson on the correct procedure for solving equivalence problems. All students were given a conceptual assessment and a procedural assessment that included standard problems (which were in a consistent format across the entire study) as well as transfer problems (which varied features of the problem like the operation used or the position of the blank in the equation).
The researchers found that “conceptual instruction led to increased conceptual understanding and to generation and transfer of a correct procedure. Procedural instruction led to increased conceptual understanding and to execution, but only limited transfer, of the instructed procedure” (Rittle-Johnson and Alibali 1999). In other words, students who received the conceptual instruction were able to apply their knowledge more flexibly to novel problems.
-
Conceptual and procedural knowledge are mutually reinforcing, but conceptual knowledge generally provides a stronger foundation for student learning. In a 2015 review of available research, Dr. Bethany Rittle-Johnson and Dr. Michael Schneider found that the relationship between conceptual and procedural knowledge is bidirectional, with increases in conceptual knowledge leading to increases in procedural knowledge and vice versa. However, they also found that this relationship is not always even—conceptual knowledge more strongly and consistently supports procedural knowledge than the reverse.
The National Council of Teachers of Mathematics (NCTM) also supports these findings, stating that “effective teaching of mathematics builds fluency with procedures on a foundation of conceptual understanding so that students, over time, become skillful in using procedures flexibly as they solve contextual and mathematical problems” (NCTM 2014; italics added).
-
Increased conceptual knowledge may reduce students’ math anxiety. In 2017, researchers looked at the relationship between conceptual knowledge and math anxiety in a sample of remedial math students from four elementary algebra sections at an urban community college. In two of the four sections, instructors followed lesson plans focused on concepts, while the other two sections received instruction focused on procedures. Participants completed the Mathematics Anxiety Rating Scale at the beginning and end of the courses. The researchers found that students in the concept-based course had better conceptual understanding of the content, performed better on the given procedural quiz, and had a much greater improvement in math anxiety scores than students in the procedure-based course (Khoule, Bonsu, and El Houari 2017).
The research is clear: Memorization is an integral part of learning math, and becoming fluent with core math facts and concepts requires it. What many educators have failed to do for so long, however, is give students the opportunity to memorize with meaning. Helping students develop an underlying understanding of math concepts will enable them to be successful with increasingly abstract and complex concepts and their varied applications.
Conceptual knowledge allows students to form a flexible, coherent body of math knowledge and provides a foundation for connecting new ideas to what they already know. And this has benefits outside math class as well—from recognizing rhythmic structures in music to interpreting statistics in a science project—as students apply and adapt their knowledge.
Two Ways That Math Instruction Falls Short on Knowledge Building
Giving students a specific procedure that they can apply easily in the moment to a set of problems is a familiar approach to math instruction. The idea is that students will become quick and efficient in their execution, and with sufficient practice with procedures, deeper understanding will eventually follow.
But learning skills without a foundation of understanding is more difficult for students, and they’re also more likely to make errors and forget what they’ve learned. Students who forget a procedure will often get stuck on problems, while those who understand underlying concepts can reason and use a variety of strategies to complete them.
Unfortunately, two common barriers prevent students from developing a strong foundation of conceptual knowledge in today’s math classrooms.
-
The use of disjointed math curricula. Researchers from the Third International Mathematics and Science Study (TIMMS) found that coherent curriculum is the primary predictor of student achievement in math and science (Schmidt, Wang, and McKnight 2005). Yet K–12 math curricula often cover a long list of seemingly separate topics that are chopped up into discrete chunks aligned with typical standardized testing assessments.
Most curricula ignore how math skills connect to one another and how concepts layer over time and intentionally scaffold students to more advanced topics. Curricular materials too often consist of isolated exercise sets that make it difficult for students to develop deep, enduring math knowledge and the problem-solving abilities they need for proficiency and long-term success in math class and beyond.
-
Leading with and relying on math shortcuts. From PEMDAS for the order of operations to the butterfly method of adding fractions to multiplying binomials with FOIL, there is a long-standing tradition of using mnemonic devices, tricks, and shortcuts in math instruction. These shortcuts can be effective tools for short-term recall, but they can quickly become a means to an end—if correctly applied, the trick results in the right answer.
The breakdown comes when students focus on tricks and shortcuts without
an understanding of why a shortcut or memory trick works (and when those techniques may fail). While mnemonics may support memory, they alone will not support learning. Absent conceptual understanding, students may struggle when they encounter new or more difficult problems. As cognitive scientist Daniel Willingham (2006) explains:Willingham points to a large body of research that shows that novices focus on the surface features of a problem, whereas those with more knowledge see and approach problems differently because they focus on key underlying structures.
The student who does not have the distributive property firmly in memory must think it through every time he encounters a(b + c), but the student who does, circumvents this process. Your cognitive system would indeed be poor if this were not possible. The challenge, of course, is that you don’t always see the same problem, and you may not recognize that a new problem is analogous to one you’ve seen before .... Fortunately, knowledge helps with this.
How do these barriers affect student achievement? National Assessment of Educational Progress (NAEP) data reveal that the percentage of students at or above proficiency in math has remained relatively unchanged in the last decade. Although 41 percent of grade 4 students performed at or above the proficient level in 2019, that number drops to 34 percent for grade 8 students and to just 24 percent for grade 12 students (NCES 2019). Clearly, we must urgently consider how we can better support students in developing math knowledge that endures and builds year over year.
Four Ways to Build Conceptual Knowledge in Your Math Classroom
Definitions of conceptual learning can be conflicting and vague, even after decades of discussion. Some interpretations—like discovery math—have attempted to turn math instruction completely on its head by having students use their own exploration and deduction abilities to solve problems and build conceptual understanding. But in practice, this type of approach has often resulted in more confusion for students and families, and research suggests that discovery-based instructional methods alone are less effective than a blend of explicit and discovery-based methods (Bryant et al. 2017).
There is no roadmap for balancing conceptual understanding and procedural fluency in math instruction and, as a result, educators may have difficulty picturing what effective conceptual learning is and what it looks like in practice. Here are four ways to promote deep conceptual understanding in your math classroom.
- Implement a high-quality math curriculum that promotes coherence across topics and grade levels.
Current practice: Math instruction often favors procedural fluency, and common curricula promote unfocused, disjointed, and shallow exploration of math concepts within and across grade levels.
Research in action: NCTM (2007) finds that “students taught using a standards-based curriculum, compared with those taught using more conventional curricula, generally exhibited greater conceptual understanding and performed at higher levels with respect to problem solving... . These gains did not appear to come at the expense of those aspects of mathematics measured on more traditional standardized tests” (1).
NCTM (2000) notes three features that distinguish a high-quality, coherent math curriculum. First, it explicitly links mathematical concepts, procedures, and ideas so that they build on one another and systematically expand and deepen students’ capabilities. Second, it focuses on math concepts that enable students to solve problems in a variety of important, real-life settings (including school, home, and work). And third, it prepares students for continued study across grade levels and challenges students to learn increasingly more sophisticated mathematical ideas.
Students who are not exposed early on to a coherent knowledge-building math curriculum lose out on opportunities to internalize a network of relevant math concepts, setting students up for deeper engagement with math as they progress through school.
-
Provide ample opportunity for students to talk about math.
Current practice: Most math classrooms feature a teacher lecturing about a particular concept or demonstrating a procedure and students quietly working on practice problems.Research in action: NCTM (2014) identifies math talk, or “facilitating meaningful mathematical discourse,” as one of eight research-informed actions included in high- quality math education (3). Researchers have found that math talk supports student learning. It can improve memory and understanding, aid the development of language and social skills, and boost confidence and interest in math, particularly discourse that is “academically productive ‘in that it supports the development of students’ reasoning and students’ abilities to express their thoughts clearly’” (NCTM 2013, 4).
When students engage in authentic and rigorous math tasks and then talk about their strategy choices and solution reasoning, they have the opportunity to organize and clarify their understanding, see processes and ideas from multiple perspectives, and refine their mathematical thinking. Some strategies that promote math talk in the classroom include asking questions to build understanding, having students turn and talk in pairs or small groups, and debriefing lessons at their conclusion.
-
Deepen conceptual understanding with multiple representations.
Current practice: Lessons often present students with one right way to approach and solve a problem, and when multiple representations of a problem are used there is not enough focus on the relations or connections between them.Research in action: Any given math problem can be represented several different ways, and it is often difficult for one single representation to show all aspects of a math concept. “Using and connecting mathematical representations” is another of NCTM’s (2014) eight research-informed actions for math education (3). Using multiple representations can give students different access points to a concept, provide opportunities for reasoning and flexible problem solving, and greatly benefit student learning.
Use and Connect Mathematical Representations
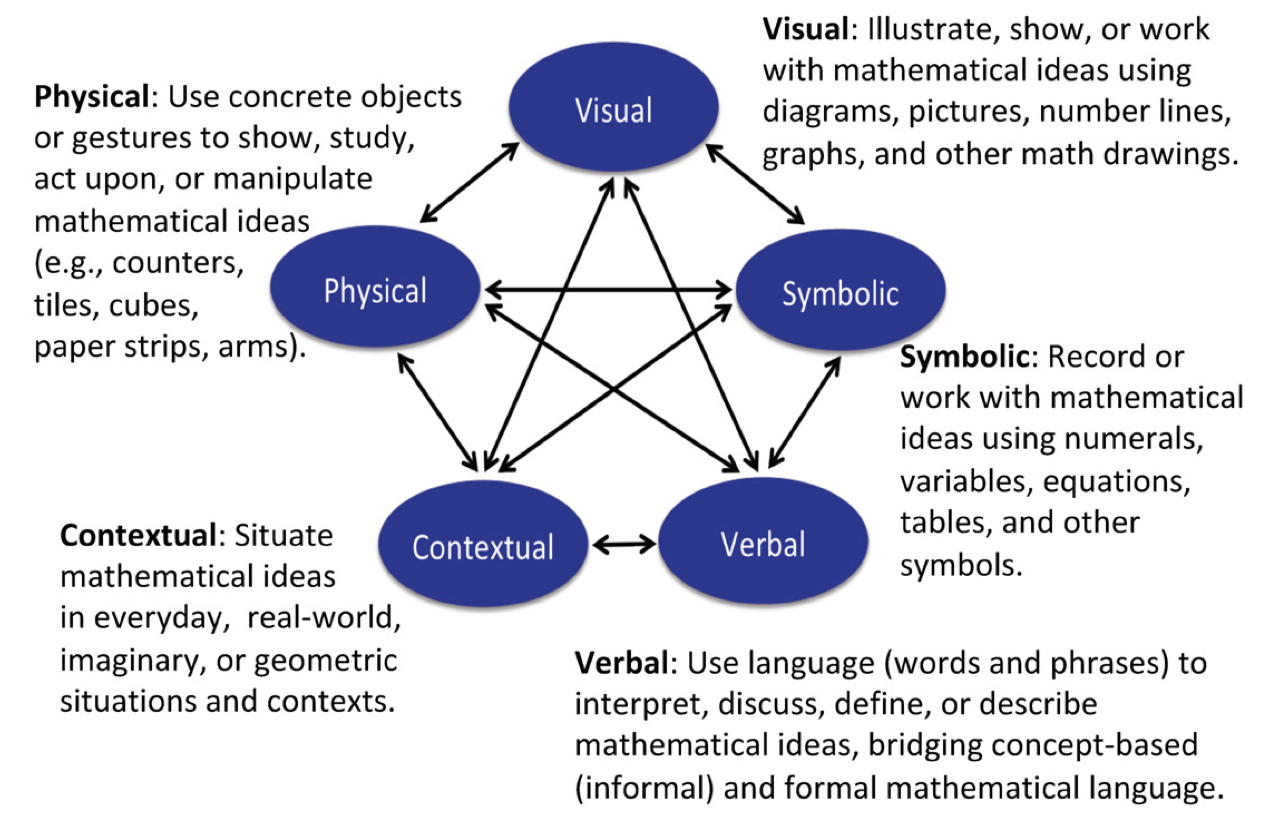
(Huinker 2019)
Some research cautions that multiple representations may confuse students if they cannot accurately interpret each individual representation and make connections among different representations and the information they convey (Rau and Matthews 2017). But multiple representations that are blended into a deliberate sequence—like the concrete–pictorial–abstract progression—are an effective way to lead students to a deeper and richer understanding of abstract concepts. Prompting students to reflect on and discuss different representations as they work on problems will also enable them to connect different forms, better grasp key underlying ideas, and deepen their understanding of how to appropriately apply different representations and strategies to do math. - Encourage students to identify, define, and create patterns and relationships.
Current practice: The development of students’ pattern awareness is left to chance as students lack sustained and explicit attention to patterns across math concepts and grade levels.
Research in action: Children become aware of patterns at a very early age—they are attuned to repetitive daily routines, rhythmic patterns in songs and language, and shape patterns with blocks and toys. Counting itself is a simple pattern: increase by one.
The structure of math is built around looking for and manipulating patterns, and many major concepts in algebra and geometry are, at their core, generalizations of patterns in number and shape. In a study of children ages 4 to 11, Dr. Bethany Rittle- Johnson and colleagues found that young children’s ability to spot mathematical patterns was a unique predictor of later math achievement, a better predictor than other math and nonmath skills such as counting (Rittle-Johnson et al. 2016).
Not all students will naturally pay attention to patterns. Making use of problem sequences and patterns in problem sets, explicitly calling out patterns during instruction, and posing questions that encourage students to spot underlying patterns can help students identify mathematical relationships and build conceptual understanding. Students who are comfortable looking for, analyzing, and generalizing patterns can use those skills to break down complex problems and integrate new concepts.
It is possible to help students gain deep, enduring, and practical understanding of math concepts alongside procedural skills and fluency. Armed with both, students can become confident and proficient with math inside and outside the classroom. Click here for more detail on these strategies in action.
Download the Article as a Free PDF
Alyssa Buccella
Alyssa has nearly a decade of education research experience. She has led equity and student success research to support K-12 public school districts across the country in addressing their most pressing challenges, including college access, mental health, social emotional learning, and racial justice. Alyssa holds a B.A. in Psychology and Global Studies and an M.Ed. in Globalization and Educational Change from Lehigh University.
Topics: math
Get the Latest Great Minds Updates
Stay informed of simple yet crucial implementation support tips, curriculum updates, new resources, professional development opportunities, and more for math, science, and ELA.
