Posted in: Aha! Blog > Eureka Math Blog > Implementation Support > Three Steps for Acing Pacing in a Lesson
This blog entry is a follow-up to our Eureka Math pacing webinar series from October 2017. It focuses on the process of planning the specific content of a 60-minute math lesson, which in turn helps teachers stay on pace. You can view an on-demand version of that webinar, “Acing Pacing: Tools and Strategies to Maximize Instructional Time,” here.
Our “Acing Pacing” webinar series focused on tools and strategies that help educators maximize instructional time at the module level, but many attendees were interested in further thoughts on “acing pacing” at the lesson level. The key to successful pacing of each day’s lesson is to plan a topic at a time. When reviewing and planning all the lessons within one topic together, teachers can more clearly identify the elements they will need and the elements that they might choose to leave out for each particular lesson.
Focusing on the Topic First
Starting at the topic level can be the key to alleviating the stress of pacing at the faster-paced lesson level. With a clear understanding of the topic’s goal, teachers can adjust lessons from one day to the next, even if that means they may not “get to everything” in the way they first planned. We strongly recommend planning per topic in your next round of preparation; see how that helps clarify teaching focus during each day’s lesson. Let’s use Grade 2 Module 5 Lesson 9 (G2 M5 L9) as an example of preparing to plan a lesson with the topic’s goal in mind.
To ace pacing of lessons, consider the same three aspects that we discussed in the webinar for pacing at the module level: the time available, the progression of learning, and the students. For this blog, we’ll assume teachers have 60 minutes allotted for math.
Step 1: Discern the Plot
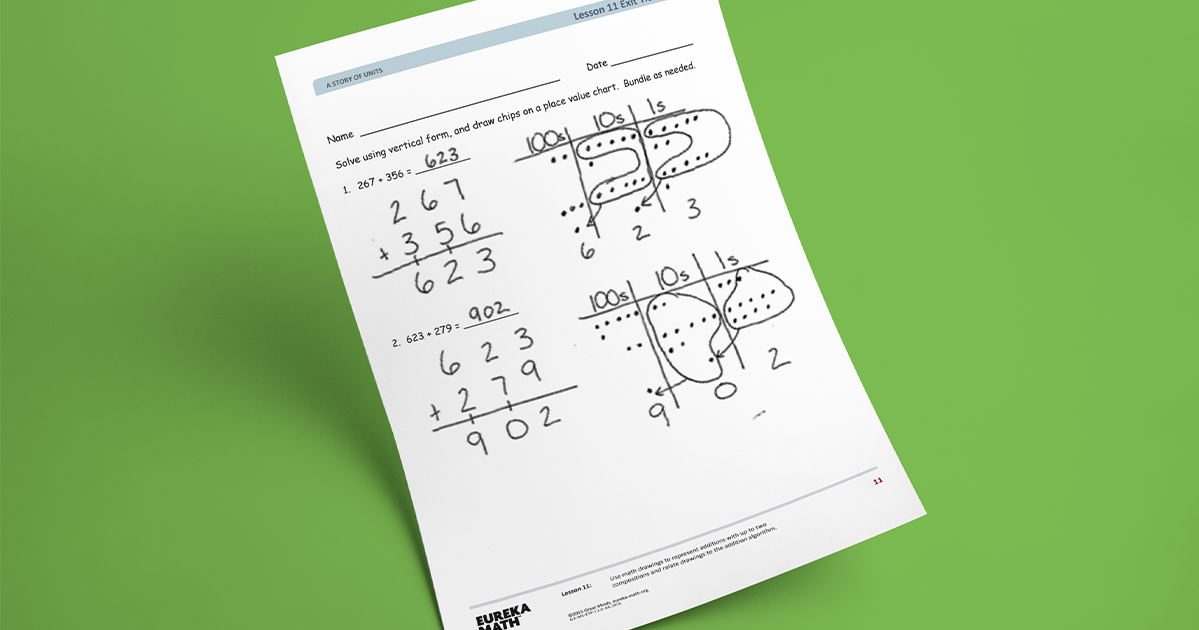
The webinar outlines a brief process of discerning the plot for G2 M5. Now let’s focus on Topic B, Strategies for Composing Tens and Hundreds within 1,000, which includes the lesson we are preparing, Lesson 9. First, we analyze the Table of Contents, Module Overview, and Topic Overview. Next, we study the Exit Tickets, shown below, because they give a quick snapshot of what student learning should look like each day. Looking at all the lesson’s Exit Tickets at once reveals the trajectory of the mathematics in the lessons.





To plan a specific lesson, such as Lesson 9, work backward by starting with the Exit Ticket to make sure you have a clear sense of the goal for the day. After completing and analyzing the Exit Ticket, you know your goal is to ensure that students understand how to handle having more than 9 of a given unit, how to compose 10 tens to make a new hundred, and how to compose 10 ones to make a new ten. You also want students to take ownership of their learning by using place value language to explain their thinking and problem-solving processes. Students had many experiences composing a new ten and a new hundred during Module 4. Your goal is to connect their models with the vertical form’s symbols.
Finally, reviewing the assessment is an important part of discerning the plot. Specifically, consider how the assessment addresses the topic objectives.
Step 2: Find the Ladder
Now that you’ve studied the plot of Lesson 9, it’s time to look deeper into the lesson itself. Start with the Problem Set. The Problem Set shows a roadmap of the work in each lesson.
Step 2 is called Find the Ladder because Problem Sets are written as “ladders.” The first problems are like the first rungs. They’re intentionally the simplest rungs to reach so that most students can step up and get started. Moving through the Problem Set is like moving up the rungs of a ladder; step by step the complexity increases as students strive toward the lesson’s objective. As you study Problem Sets, you might also find that the final problems are a stretch, designed for students who are up to the challenge of moving beyond the objective. That’s intentional.
Begin by doing the Lesson 9 Problem Set yourself and carefully analyzing its movement from simple to complex. Refer to the lesson as necessary to see how students are expected to solve the problems.
As you complete and analyze the simple-to-complex sequence, here’s what you’ll notice in Problem 1.

Problem 1
a. Two compositions
b. Two compositions
c. Two compositions
d. Two compositions
e. No compositions; Note in Debrief about relationship to 1(f)
f. No compositions; Note in Debrief about relationship to 1(e)
g. Two compositions; Final composition adds to thousands place
h. Two compositions
Problem 2 has a series of problems with directions that offer a choice of strategies for solving. Here’s what you will notice when you complete and analyze the simple-to-complex sequence.

Problem 2
Students can solve problems any way. This includes making the next hundred or making the next ten as they add. Along with the vertical form, students may use number bonds or the arrow way to show their thinking.
Opportunities for specific combinations are:
a. 7 tens and 3 tens make the next hundred
b. 6 tens and 4 tens make the next hundred (slightly more difficult pair)
c. 4 tens 5 ones needed to make the next hundred, or 5 ones need 5 more ones to make the next ten (doubles fact)
d. 4 tens and 2 ones needed to make next hundred
e. 11 more than 700; Adding like units (hundreds to hundreds and ones to ones) may be most efficient; arrow way may be useful in clearly demonstrating varied ways to add 303
f. Use 2(e) to solve; 478 is 70 more than 408 in 2(e)
g. Use 2(f) to solve; 323 is 20 more than 303 in 2(f); 2(d) through 2(g) all have 8 in the ones place of the first addend and 3 in the ones place

Lesson 9 Debrief
You may think your students won’t notice that they can use Problem 2(e) to help them with Problem 2(f). You’ll probably be right about that at first; they may not notice the similarities without some coaching.
That’s where the Student Debrief comes into play. It is our responsibility as mathematics teachers to foster students’ content knowledge through metacognition. In other words, we need to provide students with opportunities to think about and practice mathematical thinking so they get in the habit of looking for and noticing connections like the ones we see in this Problem Set. The daily Student Debriefs in Eureka Math lessons are intended to do just that.
When crafting the Problem Sets, the writers took notes about the various connections, patterns, and other details that teachers might want to bring to students’ attention. They wove these items into daily lessons as suggested questions or talking points in the Student Debrief.
As you move through step 2, Find the Ladder, look at the Lesson 9 Debrief, paying special attention to the bulleted items (pictured). Ask yourself the following questions:
What does the Debrief emphasize from the Problem Set?
Why might the writers have chosen to include those points instead of others?

Before honing the lesson in step 3, look at the Problem Set and anticipate where students may have difficulty with the content. Also, think about questions or talking points you could use in the Debrief to support students through struggles with content or with developing metacognitive skills. For instance, with my own class, I chose to focus our Student Debrief conversation on the second and fourth bullets. I place notes on the side because I plan to use students’ work on Problem 2 if possible.
Step 3: Hone the Lesson
When honing the lesson, consider what you want your students to complete in the Problem Set and what learning and experiences should occur to support productive struggle followed by ultimate success. Select the problems in the Problem Set that are Must Do (M), Could Do (C), and Extension (E) problems for your students.
Let’s say that for my own class I choose Problems 1(a) through (c) and Problems 2(a) and (c) as Must Do problems for my class. I mark those with an M because I believe these reflect the goal of the Exit Ticket and objective well. Students could do the rest of the problems (I mark those with a C). I don’t think that any problems listed are extensions. I jot myself a note that students who are ready for an extension could model multiple ways to solve the same problem from Problem 2. (I mark that note with an E.)
Then, I review the Concept Development for what I want this instructional time to sound and look like. I jot notes about the main examples, language, and representations to model and write down any questions I want to make sure I ask students.



I then look briefly at the Application Problem to determine whether it builds toward the day’s objective or can be considered separately.
For Lesson 9, notice that the Application Problem is a two-step, labor-intensive word problem. In my class, I want to give this problem more time and attention, so I move it out of Lesson 9. Lesson 11 will be a better time to use this Application Problem because the Concept Development will move quickly. I can swap out Lesson 11 Application Problem and use it during Lesson 9. The Lesson 11 Application Problem gives me a chance to see what strategies they are using independently to solve double-digit addition with a composition.
Finally, I review the Fluency Practice suggestions to determine which Fluency Practice activities address foundational concepts in today’s lesson and which contribute to maintenance of general skills. Depending on my time frame and my students’ areas of need and strength, I can choose which Fluency Practices to include, to extend, or to leave for another day.
Three Fluency Practices are allotted for the day. All of them can support today’s lesson in some way, but the first Fluency Practice, Making the Next Ten to Add, will most benefit my students and their needs. I order the Fluency Practices in the event I have time for more than one.


When you are comfortable with organizing and facilitating the hour of instruction, use a 5ʺ x 8ʺ index card to capture the flow and major moves; use the card as a reminder during actual instruction. The card can also include timing if helpful. For instance, when I completed this lesson in my own class, I set the time I wanted to start the Concept Development portion of my lesson. I could adjust the length of the Fluency Practice based on the time left after the Application Problem without sacrificing time from the Concept Development. View an example of Lesson Customization and download my Lesson Customization Note-Taking Template here.
Final Thoughts
Planning one topic at a time gives you the opportunity to consider what came before in the module, where you are now, and where you’re going as well as the main objective of a series of lessons. By discerning the plot of the topic, you are better able to find the ladder of learning in the lesson and hone the lesson to meet the needs of students and support them along their continuum of learning during the 60-minute block of time. This lesson preparation process can be lengthy in the beginning, but it gets faster the more you work through the process, know the content, and know your students.
You can also plan with colleagues, discerning the plot together and then each working through one lesson in the topic before you reconvene. The team experience supports everyone’s understanding, learning, and decision making.
If you would like to learn more about this three-step process or want to spend a day working through the process with colleagues and Eureka Math educators, Great Minds offers a two-day Professional Development session that focuses on preparation and customization. Click here to find out more.
Submit the Form to Print

Marianne Strayton
Topics: Implementation Support