High-Leverage Teaching Practices that Positively Impact Student Learning

Whether you’re a veteran math educator looking to refresh your skills or a new classroom teacher, we’ve assembled a collection of teaching practices that you can easily use in the implementation of Eureka Math²®.  Research published in 2014 by the National Council for Teachers of Mathematics (NCTM) determined that these eight high-leverage teaching practices enable students to develop metacognitive skills as they learn mathematical concepts and procedures through meaningful tasks and the right discourse (NCTM, p. 10). These practices form the foundation of successful math teaching and learning (NCTM, p. 10). Rather than viewing these practices as a checklist of strategies, challenge yourself to see the purpose and value of each practice. In this post, we’ll explain how they all interact with one another within a single math lesson and across many modules of learning of Eureka Math².  

Math Teaching Practice 1: Establish mathematical goals to focus learning. 

Eureka Math² lesson objectives concisely state the learning that students will engage with during the lesson. An example of how student progress toward the lesson objectives is provided by the Exit Ticket shown in the lesson overview.

Each lesson also includes one or more objective-aligned Key Questions. The answers to each question are developed throughout the lesson, and students respond to each Key Question during the Debrief segment at the end of the lesson. Achievement Descriptors (ADs), tagged to each lesson and assessment item, are standards-aligned descriptors of what students should know and be able to do at the culmination of each module. Each AD has a rubric with Proficiency Indicators that use sample items to clarify for you what proficiency looks and sounds like.  

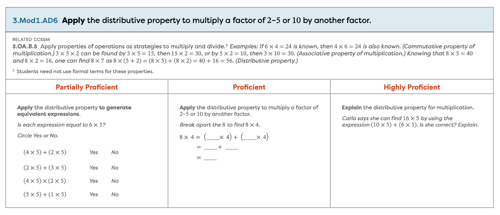
Module and Topic Overviews as well as the Why section also provide illustrated narratives of the mathematical learning goals and progressions. All of these Eureka Math² features emphasize the mathematical goals of each lesson so that you’re always focused on what your students should be learning.

Math Teaching Practice 2: Implement tasks that promote reasoning and problem solving. 

Great Minds® agrees that “Regular opportunities for each and every student to engage in high-level tasks, which promote reasoning and problem-solving, provide multiple entry points (low floor), and encourage use and discussion of varied solution strategies (high ceiling), are an important step toward establishing and enhancing students’ positive identities and sense of agency in mathematics” (Huinker and Bill, 2017, p. 64).  

Eureka Math² lessons engage all students with low-floor, high-ceiling tasks; word problems of varying types and complexities; and real-world problems that promote reasoning and problem solving. Many lessons also include open-middle and open-ended tasks that engage students in problem solving. Open-middle tasks allow you to pose a math task that is common to all students, yet students can utilize any strategies to arrive at a common answer. Similarly, open-ended tasks pose a common starting point for all students, usually one that is mathematically approachable for everyone. From there, the strategies your students use and the solutions they arrive at can vary. This approach to lesson design allows students to demonstrate their mathematical knowledge and establish their positive identities in multiple ways, such as guided whole-group discourse, partner discussions, collaborative instructional routines, and share–compare–connect segments. When students learn reasoning and problem solving through engagement with rich tasks and multiple means of expressing their knowledge, they build positive identities as capable, confident mathematicians. 

Math Teaching Practice 3: Use and connect mathematical representations. 

Eureka Math² includes coherent, high-leverage physical and digital mathematical representations across the curriculum, such as cubes, place value disks, number bonds, number lines, and tape diagrams. Students encounter the same representations applied to similar concepts across grades. That familiarity makes it easier for them to connect concepts together and accelerates the pace of your instructional time.

Intentionally designed lessons in Eureka Math² provide relevant problem-solving contexts, including many opportunities for students to discuss their thinking. Students are taught to represent these contexts and their solution pathways symbolically, such as with equations, tables, and graphs, and then they are encouraged to select their own representations to solve problems. As they use and discuss various representations to solve a problem, students naturally make connections and comparisons that deepen their conceptual understanding.

Math Teaching Practice 4: Facilitate meaningful mathematical discourse. 

Eureka Math² intentionally facilitates authentic, meaningful mathematical discourse in each lesson, which starts with the belief that all students’ ideas and questions are valuable. Implementing this belief requires creating a classroom environment where all students feel comfortable taking risks, making mistakes, talking, and learning from one another. This environment fosters community, allows students to express mathematical ideas, and positively affects participation and engagement. 

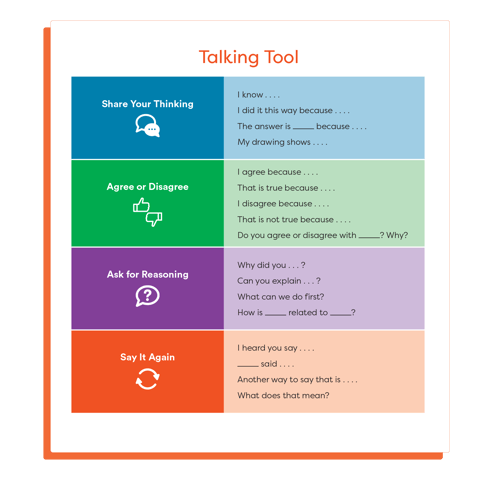
Our lessons facilitate discourse through context videos, digital interactives, instructional routines, partner and group work, and classroom discussions supported by a Talking Tool. These activities are designed to help students build a shared understanding of mathematical ideas by analyzing and comparing student approaches and arguments.

Math Teaching Practice 5: Pose purposeful questions. 

The lesson vignettes in Eureka Math² include carefully crafted questions that assess and advance students’ reasoning and sense-making about important mathematical ideas and relationships. These questions vary in purpose: gathering information, probing thinking, and making the math visible, which encourages reflection and justification. Sample student responses show teachers how the answers to these powerful questions can connect with follow-up questions to drive deeper understanding. Margin boxes containing questions designed to engage students in the Standards for Mathematical Practices are placed at the point of use in each lesson. Students are also encouraged to formulate and ask questions by using the sentence starters and frames in the Talking Tool.

Math Teaching Practice 6: Build procedural fluency from conceptual understanding. 

Problem sequences, models, and discussion questions in Eureka Math² are designed to help students develop a conceptual understanding of key mathematical strategies while building procedural fluency throughout the modules. Students understand the concepts through concrete, pictorial, and abstract representations that are presented in a careful progression across lessons. 

After the conceptual foundation is in place, students continue to practice and draw upon their learning as they solve contextual and mathematical problems. Over time, students are encouraged to flexibly use a variety of tools and strategies as they apply the concepts in increasingly challenging problems, making them more efficient with their procedural skills. In addition, the Remember problems and daily Fluency section in Eureka Math² lessons provide ongoing, systematic distributed practice that supports students in attaining the required fluencies. 

Math Teaching Practice 7: Support productive struggle in learning mathematics. 

Lessons in Eureka Math² intentionally support productive struggle by inviting children to follow their mathematical curiosity and collaborate with their peers as they grapple with rigorous and relevant problems. To keep that struggle focused on mathematical concepts, accessibility is at the forefront of our lesson design.

Instructional routines such as Math Chat and Take a Stand—along with real-life, wordless context videos and digital interactives—make rigorous problems accessible to all. Careful attention to readability in student-facing directions and word problems ensures that the words do not get in the way of the mathematics. Strategically placed margin notes in lessons support all students in struggling productively with mathematical ideas and relationships. These margin notes include Universal Design for Learning; Differentiation: Challenge; Differentiation: Support; Language Support; and Promoting the Standards for Mathematical Practices. Additionally, students are invited to engage in mathematics and build their knowledge through Math Past and fine art connections. 

Math Teaching Practice 8: Elicit and use evidence of student thinking. 

In addition to discussion-based lessons focused on your students’ work, Eureka Math² provides visibility into student learning and thinking through a comprehensive assessment system that includes formative, summative, and diagnostic assessments that generate data from many perspectives. The Eureka Math² Equip™ Pre-Module Assessments help you see student thinking about the essential foundational concepts in the modules that you are about to begin. The standards-aligned ADs give you a framework for understanding what your students’ thinking should look like after engaging in the learning of the module.

Assessments also track your students’ thinking in different forms throughout a module. Exit Tickets and Topic Quizzes are formative assessments designed to give teachers actionable data about student thinking to inform instruction in Grade Level 3–Algebra I*. Observational Assessment Recording Sheets help teachers in Grade Levels PK–2 collect and track student progress. In all grade levels, Module Assessments measure student understanding after each module and can be delivered on paper or digitally. Finally, Benchmark Assessments are summative assessments that are given three times a year in Grade Level 1–Algebra I* and provide a wider view of student thinking.

Every component of Eureka Math² is thoughtfully designed to support these Math Teaching Practices so that all of your students can learn mathematics deeply and equitably. 

*A Mathematics I integrated math course is also available.