The CASEL Framework in Action: How Eureka Math Squared Integrates Social, Emotional, and Academic Learning

Students’ social and emotional well-being is intimately connected to their academic success. Success in mathematics requires more than developing students’ mathematical knowledge. It also requires developing key social-emotional skills, such as how to communicate effectively with others, how to organize and manage tasks, and how to recover from setbacks or failures.¹

Students develop beliefs about themselves as learners of mathematics from their learning experiences, interactions with teachers, family members, and peers, as well as messages they receive from media sources. The beliefs students develop from various inputs shape their mathematical identity.² As students continue through school, their mathematical identity is either strengthened or damaged by subsequent experiences with mathematics.³

Students must also feel a part of a learning community to fully engage in the work. In classroom and school environments, social and emotional well-being requires that students feel a sense of belonging and have a measure of control over their learning.⁴ A student’s identity as a mathematics learner affects their willingness and ability to engage in the classroom community. Individually, each student should feel valued and appreciated, and they should also value and appreciate every one of their peers in the classroom—building a community of mathematics learners.⁵

Academic curricula play a central role in the development of social-emotional competencies. In mathematics classrooms, the curriculum allows for teachers and students to interact in a variety of ways.

The teacher’s role in students’ academic, social, and emotional development is complex. A teacher needs to:

• engage students in tasks that promote mathematical reasoning and problem solving,
• provide supports as needed so that all students can access grade-level math content,
• facilitate instruction that provides multiple entry points,
• value multiple perspectives and contributions,
• promote student-to-student discussion of mathematical ideas, and
• help to strengthen students’ mathematical identities as well as promote a productive classroom community.

An effective curriculum supports teachers in this work in three ways. First, the curriculum provides open-ended tasks and scenarios that create space for students to generate, test, share, and refine their ideas. Second, the curriculum builds content and language sequentially so it is easier for students to relate new learning with prior knowledge. Third, the curriculum employs routines that encourage productive student-to-student interactions when engaging in tasks and discussions.

The students’ role is to engage in tasks, activities, and exercises that are intended to develop their mathematical understanding, participate in discussions with diverse groups to explain their thinking and reasoning and to consider and critique the thinking and reasoning of others, and reflect on and adjust their participation and effort in the classroom based on feedback. An effective curriculum can support students in this work by (1) regularly providing tasks that promote reasoning and problem solving to help them make sense of new mathematical ideas and allow them opportunities to learn through productive struggle, (2) providing multiple opportunities to discuss mathematical ideas with diverse groups of peers in a variety of ways, and (3) explicitly calling out points in lessons where they might pause to reflect and receive feedback to help them monitor their own progress over time.

This document describes how Eureka Math² integrates social-emotional learning in the following sections:

• Overview: an overview of SEL integration that defines the social-emotional core competencies, the effective mathematics teaching practices, and the Standards for Mathematical Practice and explains how all three were integrated during the writing of Eureka Math².
• Elaboration: a detailed table that outlines the integration of the mathematics teaching practices, Standards for Mathematical Practice, and the social-emotional core competencies within specific curriculum materials with the dual goals of developing students’ mathematical knowledge and social-emotional skills.
• Lesson Examples: sample lessons from grades K–5 and 6–Algebra I, annotated to illustrate SEL integration to help teachers know what to look for when studying lessons and preparing for instruction.
• Additional Resources: examples of resources embedded across grade levels explicitly aligned with the SEL core competencies and the Standards for Mathematical Practice.
• Family and Community Connections: a description of the parent and community resources available in the Eureka Math² curriculum.

Overview

“A mathematics curriculum is enhanced when it is intentional about developing social and emotional learning (SEL) core competencies" (CASEL 2017).⁶ When curriculum and instruction explicitly promote all five core competencies, academic achievement is significantly increased, attitudes and behaviors improve, and emotional distress is reduced (Durlak et al., 2011).⁷

Eureka Math² is a comprehensive math program built on the research-based foundational idea that math is best understood as an unfolding story where students learn by connecting new learning to prior knowledge. Coherence across lessons, modules, and grades, consistent math models, and content that engages students in rigorous learning provide entry points for all students to access grade-level content. The result allows teachers to systematically develop concepts, skills, models, and discipline-specific language while developing students’ social and emotional competencies.

Research has demonstrated the powerful and lasting effects of integrating academic, social, and emotional learning.⁸ Indeed, such integration is key to the success of Eureka Math². Students thrive because they have the pedagogical, social, and emotional support needed for rigorous mathematics learning. Moreover, with a strong foundation of social, emotional, and academic competence, students are prepared to succeed beyond their schooling.

Eureka Math² fosters the development of the five social-emotional competencies identified by the Collaborative for Academic, Social, and Emotional Learning (CASEL) through the interaction of eight effective teaching practices (NCTM 2014) and eight Standards for Mathematical Practice (NGA Center and CCSSO 2010).⁹ The lists below provide descriptions of these competencies, teaching practices, and mathematical practices.

 Social-Emotional Competencies

1. Self-awareness. The ability to recognize one’s own emotions, thoughts, and values and how they influence behavior; the ability to accurately assess one’s own strengths and limitations, with a well-grounded sense of confidence, optimism, and a “growth mindset.”
2. Self-management. The ability to successfully regulate one’s own emotions, thoughts, and behaviors in different situations, effectively managing stress, controlling impulses, and motivating oneself; the ability to set and work toward personal and academic goals.
3. Social awareness. The ability to take the perspective of and empathize with others, including those from diverse backgrounds and cultures; the ability to understand social and ethical norms for behavior and to recognize family, school, and community resources and support.
4. Relationship skills. The ability to establish and maintain healthy and rewarding relationships with diverse individuals and groups; the ability to communicate clearly, listen well, cooperate with others, resist inappropriate social pressure, negotiate conflict constructively, and seek and offer help when needed.
5. Responsible decision-making. The ability to make constructive choices about personal behavior and social interactions based on ethical standards, safety concerns, and social norms; the realistic evaluation of consequences of various actions, and consideration of the well-being of oneself and others.

Source–Core Social-Emotional Competencies (https://casel.org/fundamentals-of-sel/what-is-the-casel-framework/)

The eight mathematics teaching practices provide a framework for enhancing mathematics teaching and learning, and they are closely related to the core SEL competencies. Teachers using these critical practices will help their students acquire a deeper understanding of mathematics.

Effective Teaching Practices for Mathematics

1. Establish mathematics goals to focus learning. Effective teaching of mathematics establishes clear goals for the mathematics that students are learning, situates goals within learning progressions, and uses the goals to guide instructional decisions.
2. Implement tasks that promote reasoning and problem solving. Effective teaching of mathematics engages students in solving and discussing tasks that promote mathematical reasoning and problem solving and allow multiple entry points and varied solution strategies.
3. Use and connect mathematical representations. Effective teaching of mathematics engages students in making connections among mathematical representations to deepen understanding of mathematics concepts and procedures as tools for problem solving.
4. Facilitate meaningful mathematical discourse. Effective teaching of mathematics facilitates discourse among students to build shared understanding of mathematical ideas by analyzing and comparing student approaches and arguments.
5. Pose purposeful questions. Effective teaching of mathematics uses purposeful questions to assess and advance students’ reasoning and sense making about important mathematical ideas and relationships.
6. Build procedural fluency from conceptual understanding. Effective teaching of mathematics builds fluency with procedures on a foundation of conceptual understanding so that students, over time, become skillful in using procedures flexibly as they solve contextual and mathematical problems.
7. Support productive struggle in learning mathematics. Effective teaching of mathematics consistently provides students, individually and collectively, with opportunities and supports to engage as they grapple with mathematical ideas and relationships.
8. Elicit and use evidence of student thinking. Effective mathematics teaching uses evidence of student thinking to assess progress toward mathematical understanding and to adjust instruction continually in ways that support and extend learning.

Source–Eight Effective Mathematics Teaching Practices (NCTM 2014, p. 10)

The eight Standards for Mathematical Practice (MP) describe ways in which students engage in and interact with the content they learn in elementary, middle, and high school. As their maturity and expertise in mathematics grows, so too should their proficiency with these practices.

Standards for Mathematical Practice

1. Make sense of problems and persevere in solving them. Mathematically proficient students explain to themselves the meaning of a problem, analyze givens and relationships, choose mathematical representations to conceptualize the situation, plan a solution pathway rather than jumping into a solution attempt, monitor and evaluate their progress, check their solutions by using multiple methods, and understand and make connections among different approaches.
2. Reason abstractly and quantitatively. Mathematically proficient students make sense of quantities and their relationships in problem situations, represent the situation symbolically, consider the units involved, attend to the meaning of the quantities within the context of the situation, and flexibly use different properties of operations to strategically solve the problem.
3. Construct viable arguments and critique the reasoning of others. Mathematically proficient students explain their thinking and justify conclusions in ways that are understandable to teachers and peers, make conjectures by building a set of logical statements, and compare two arguments for strengths and weaknesses to enhance the final argument.
4. Model with mathematics. Mathematically proficient students apply mathematics to solve problems in everyday life, identify important quantities and use tools or representations to connect their relationships, and reflect on the reasonableness of their answer based on the context of the problem.
5. Use appropriate tools strategically. Mathematically proficient students consider a variety of tools (e.g., manipulatives, estimation, technology), choose the most appropriate, and use the tool correctly to support their problem solving.
6. Attend to precision. Mathematically proficient students communicate precisely by using clear definitions and appropriate mathematical language, state accurately the meaning of symbols, and specify appropriate units and labels suitable for the problem context.
7. Look for and make use of structure. Mathematically proficient students identify and explain mathematical patterns or structures, shift viewpoints to see things as single objects or as comprised as multiple objects (e.g., see expressions in many equivalent forms), and explain why and when properties of operations are true in a particular context.
8. Look for and express regularity in repeated reasoning. Mathematically proficient students notice if patterns in calculations are repeated and use that information to solve other problems, use and justify the use of general methods or shortcuts by identifying generalizations, and self-assess as they work to see whether a strategy makes sense, checking for reasonableness prior to finalizing their answer.

Source–Standards for Mathematical Practice (NGA Center and CCSSO 2010)

The five social-emotional competencies, the eight effective mathematics teaching practices, and the eight Standards for Mathematical Practice work together to support effective student learning based on a foundation of a high-quality curriculum (Fig. 1). When teachers establish goals that are part of learning progressions and communicate the what, how, and why of those goals (Teaching Practice 1), students develop the ability to monitor their own progress toward the intended learning outcomes as well as the ability to set personal, related goals (Self-management); they are also more likely to be motivated to make sense of problems and persevere in solving them (MP1). When teachers facilitate discourse among students to build shared understanding of mathematical ideas (Teaching Practice 4), students develop the ability to consider others’ perspectives and understand social norms as well as the ability to communicate, listen well, and to seek and offer help when needed (Social awareness, Relationship skills, MP3). Finally, when teachers provide students with opportunities to engage in productive struggle and support their work (Teaching Practice 7), students learn to recognize and process their emotions and thoughts while building confidence, optimism, a growth mindset (Self-awareness), and their competence with reasoning about, modeling, and using patterns (MP.2, MP.4, MP.8).

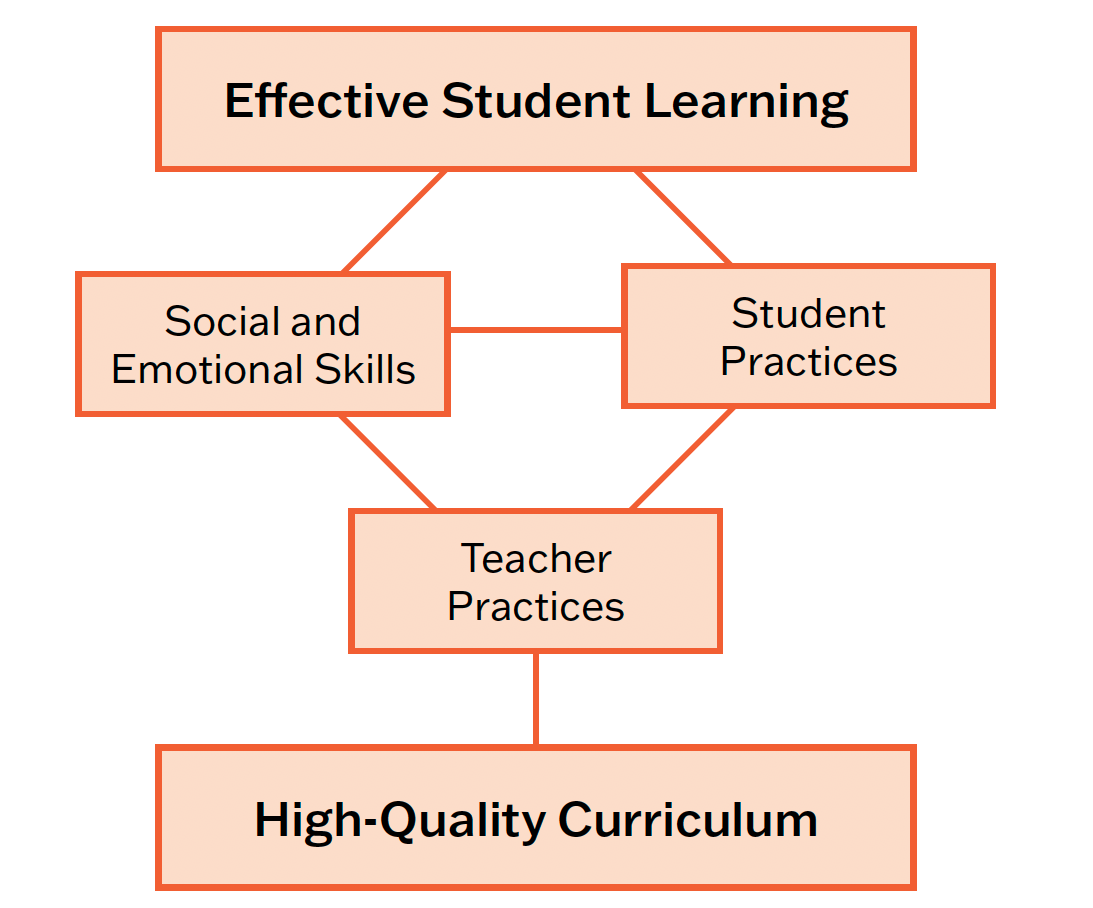
Figure 1: Effective Student Learning Supported by a High-Quality Curriculum