Coherence is a key feature of the Eureka Math2® curriculum. The problem-solving process employed in Grade Levels K–9 is a major part of that coherence. In Grade Levels K–5, students know it as the Read–Draw–Write (RDW) process. Starting in Grade Level 6 the process advances to Read, Represent, Solve, Summarize (RRSS) while maintaining the same foundational approach. Word problems become accessible as students cycle through reading and representing parts of a word problem to make sense of it. The process is simple, memorable, and powerful enough to support all students with language demands, sense-making, and algebraic thinking. This paper outlines the progression of problem-solving in Eureka Math2. We invite you to discover where your students fit into this progression, how you can support them with the work of prior grades, and build the foundation needed to problem-solve in subsequent grades.
Grade Levels K–2
The Read–Draw–Write process for solving word problems is introduced and intentionally taught step-by-step in Grade Levels K–2. You may not always recognize the developmental progression of this problem-solving process. After all, the first piece of the process is Read, a skill many of our youngest students have not learned yet. Let’s follow the journey our students take to problem-solving.
Kindergarten
One of the first experiences students have with problem-solving is counting. In the kindergarten Module 1, Topic G, Lesson 33, students use 10-frames and other problem-solving tools. Young students sometimes employ the Read-Draw-Write process without knowing it.
Are they counting a large set of objects or a small set?
Are the objects movable, or are they part of a picture?
Can they group the objects to count?
Even before students can technically read, they take in information and understand the task to get started.
The next step is Drawing. How can students organize the information they took in during the Read phase to solve the problem? For our young students, this may look like crossing out or drawing objects, it may be writing an equation or making tally marks, or it may be simply using their fingers to track their counting. Finally, students Write: they state or write the answer they have arrived at after contextualizing the problem. “There are 9 buttons!” or “I counted 25 erasers. I made 2 groups of ten and had five ones.”
Kindergarten students progress to more traditional problem-solving scenarios later in the year. In Grade Level Kindergarten Module 5 Topic A Lesson 2, students represent a math story by using pictures, number bonds, and number sentences.
There are some pigeons on our playground. Then, some more pigeons land on our playground. What could this math story look like?
This ope
n-ended math story focuses on understanding the problem and making a drawing to represent it. The “answer” will vary from student to student, emphasizing the solution path and being able to explain that path rather than getting the “right” answer. Laying the foundation for this type of thinking is important for successful problem-solving in later grades.
The title Read–Draw–Write may be misleading. Understanding and recognizing the more subtle instances of this powerful problem-solving strategy when reading, drawing, and writing may not be applicable in the literal sense is important.
Grade Level 1
In Grade Level 1, RDW expands to include all addition and subtraction problem types. Most problems students were presented with in kindergarten were the dark-shaded problem types shown in the chart. Grade Level 1 signals the transition to working with all the problem types in the chart, including the more challenging types shown in white.
As students’ problem-solving development progresses, it is important to spend time crafting the problems. Problems should be varied in type, and the numbers in the problems need to be high enough so that RDW is necessary to find the answer. For example, in Grade Level 1, Module 2, Lesson 8, students see a Change Unknown problem.
The numbers in this problem are large enough to make mental math more challenging, and the problem type lends itself to drawing to understand what the question is asking. If we ask students to use RDW with a problem that is too “easy,” for example:
Kit is coloring a rainbow.
She has 3 markers and she gets 4 more.
How many markers does she have?
Students won’t see the value in problem-solving with RDW because they could do mental math or count on their fingers, which we want them to do in circumstances like this. Teaching first-grade students to discern when to use RDW is important, and asking them to “show your work” may not always be appropriate.
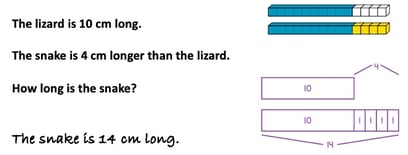
Comparison problem types frequently provide the productive struggle that leads to students seeing the need to use RDW. In Module 4, the tape diagram is taught and explored in the context of measurement. It begins with concrete cube trains (read) and moves to a tape diagram, a pictorial recording (draw), and then an equation and a statement (write).
Grade Level 2
Grade level 2 builds on the problem-solving learned in previous grades. Reading and understanding, deciding what can be drawn, and writing equations and statements to find the answer all remain the same, with the added complexity of bigger numbers, two-step problems, and more calculation strategies.
With these added problem-solving complexities, Grade Level 2 students will find the part between reading and drawing the most challenging. Drawing a model that shows what is being asked in the problem and what helps students solve the problem needs to be the focus in this teaching. For example, in Module 4 Lesson 3, this problem requires two-steps to solve it. First, a student must understand that they need to find the total number of cars and trucks. Second, they must understand that they are only solving for the number of trucks. The round numbers in this problem are intentional because we want students to attend to the set-up of the problem rather than worrying about the calculations.
Further along in the Module, the numbers and the problem types increase in complexity. The focus remains on drawing or representing the problem, so students perform the correct operation with the correct numbers. Too often, students look at the numbers presented in a word problem and guess whether they should add or subtract them to get an answer. We hope to prevent that by teaching the RDW problem-solving process, as shown below.
Grade Levels 3–5
In Grade Levels 3–5, students use the RDW process to solve word problems involving all operations and to build multi-step problems. Lessons emphasize students developing strategies to address newer complexities of multiplication, division, and working with fractions in word problems.
Beginning in Grade Level 3, students are introduced to division through the context of word problems and encounter two types of division. In a partitive division problem, the number of groups and the total are known, but the number in each group is unknown. Partitive division asks, “How many are in each group?” In measurement division, the total and number in each group are known, but the number of groups is unknown. Measurement division asks the question, “How many groups are there?” Using the RDW process, students create a drawing that accurately represents the known and unknown number of groups and the number in each group to reveal the solution path.
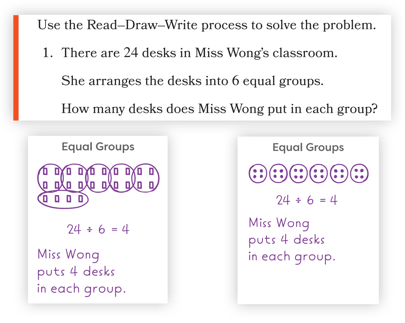
In Grade Level 3, Module 1, Topic B, Lesson 9, students solve a partitive and measurement division problem using the RDW process. As they read and draw, they discern and represent what is known. Then, they identify the unknown and use division to solve it. In the problem shown here involving 24 desks, the number of groups is known. One approach is to begin by drawing 6 circles to represent the groups, then sharing the 24 desks between the groups. There is flexibility in the RDW process for students to draw and solve in a way that makes sense. In this case, a second student starts by drawing the 24 desks and knows they must form 6 equal groups. They can determine that forming six equal groups will result in 4 desks in each group.
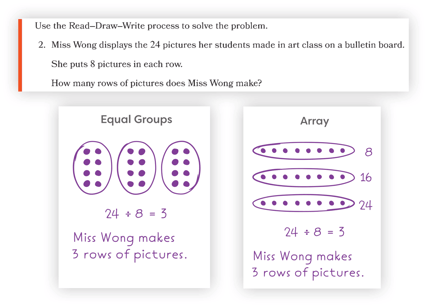The next problem, also shown here, presents a known number in each group (8 pictures in each row), and students find the unknown number of groups. Again, students can use an equal groups model. However, this time, they draw eight dots in groups or rows until they reach 24, then find the number of groups or rows. When students select their own solution strategies and decide which type of model to draw, they are using appropriate tools strategically and employing Standard for Mathematical Practice 5 (MP5). In the Lesson Debrief, students reflect on the RDW process and how identifying the known and unknown information in a problem helps them solve it. Sample responses highlight the ways a drawing can support students.
How does thinking about what is known and unknown help you
solve division word problems?Thinking about what is known helps me know if I should draw the number of groups or the number in each group.
If the unknown is the number of groups, I can count the number of groups in my drawing to solve the problem.
If the unknown is the number in each group, I can look at the number in each group in my drawing to solve the problem.
Later, in Lesson 18, students represent partitive and measurement division word problems with tape diagrams, rather than with equal groups. As a built-in feature of the RDW process, the teacher asks consistent questions: What is known? What is unknown? and How is it represented in the tape diagram? Students can consider those questions and rely on the familiar process of reading and representing the problem in chunks until identifying a path to solve it, even with the more abstract tape diagram. That process also reveals the difference between partitive and measurement division problems and deepens students’ understanding of problem-solving.
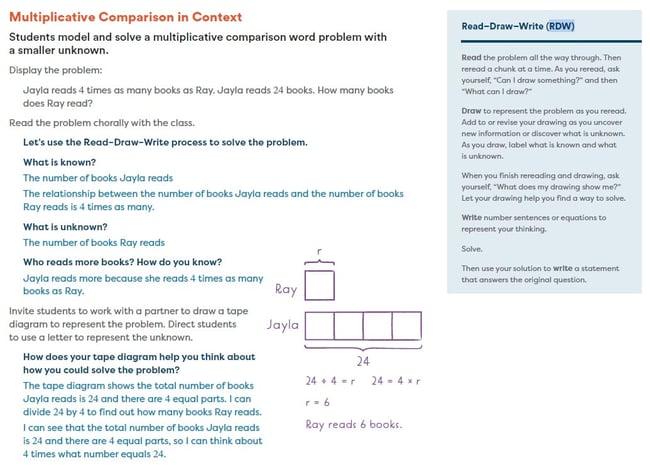
In Grade Level 4, word problem contexts expand to include multiplicative comparisons. There are multiple variations of the language in a problem that students encounter. Reading and drawing to represent the parts of the problem helps students distinguish between the known and unknown. In multiplicative comparison problems, the relationship between two quantities is often stated as “___ times as many as ___”, in other contexts, it is stated as “___ times as much as ___”, or even more specifically, “___times as heavy/long/tall as ___”. Both quantities may be given, and the total is unknown, or the total and one quantity are known, and the second quantity is unknown. Depending on the known and unknown quantities in the problems, students use multiplication or division to solve.
The RDW process supports students as they learn to interpret and evaluate multiplicative comparisons within word problems. For example, in the problem from Grade Level 4, Module 1, Topic A, Lesson 2 shown, students first Read and encounter comparison language in the phrase “4 times as many books.” Students typically represent the quantities with two separate tapes. The first tape shows 1 unit, the books that Ray reads. The second tape shows 4 units, or 4 times as many as Ray, the number of books Jayla reads. As part of the Draw step in the RDW process, students look at the tape diagram they’ve drawn and consider what it’s showing, what is known and unknown. This leads students to determine how to solve the problem. In this example, students recognize that they know the total of 4 units, so they can divide to find the unknown value of 1 unit.
How do you decide when to rename a product that Is a fraction greater than 1 as a mixed number?
If it’s a word problem, thinking about what the product represents helps me decide when I should rename fractions greater than 1 as mixed numbers. If the answer doesn’t make sense as a fraction greater than 1, then I can rename it as a mixed number.
Sometimes the question in the word problem helps me think about whether I should rename the fraction greater than 1 as a mixed number. When there isn’t a word problem or directions to write the answer as a mixed number, we can leave the product as a fraction greater than 1.
In Grade Level 4, Module 4, Lesson 33, students solve the problem: A kitten weighs 4⁄5 kilograms. A puppy is 6 times as heavy as the kitten. How many kilograms does the puppy weigh? Again, students can read the problem in chunks and draw a tape diagram to represent the weights of the kitten and puppy. The same process of reading and representing the problem supports problem-solving, even when one of the values is a fraction. However, with a fractional value, students consider whether to state their answer as a fraction greater than one or a mixed number. In the Write step, students return to the word problem to write an accurate solution statement and use the problem’s context to decide which number type makes sense to answer the question posed. As students engage in that decision-making process, they are reasoning abstractly and quantitatively and engaging in Standard for Mathematical Practice 2 (MP2). The Debrief discussion shown here emphasizes the role of the RDW process in deciding how to record an answer.
As the repertoire of problem types continues to expand along with number types, RDW continues to be a supportive process students use to read and understand a problem, create a drawing that clarifies the known, unknown, and solution path, and to state the answer in a way that makes sense.
In Grade Level 5, the complexity of word problems advances to multi-step (3+ steps) word problems. Students reiterate the RDW steps of reading a chunk and representing the information in a drawing multiple times. Students practice refining this process to create an accurate drawing and to use the RDW process efficiently in Module 3, Lessons 20 and 21.
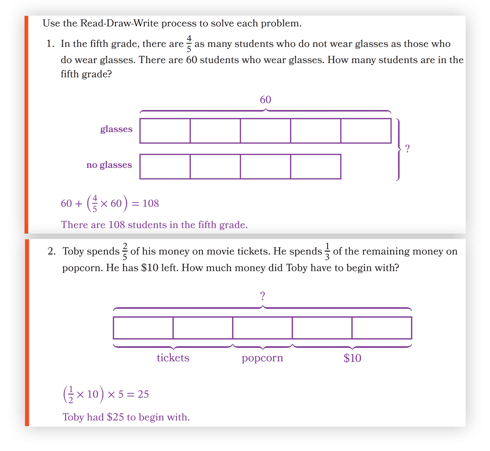
In Lesson 20, through the RDW process, students explore multiple ways fractional amounts can be represented in tape diagrams. In reading and understanding the language of the problem, students determine whether it is a comparison problem, which helps them decide how many tapes to draw. Students also represent fractional amounts such as “2⁄5 of his money” and “1⁄3 of the remaining money.” When students draw step by step as they read, they first partition the tape diagram into 5 parts and label 2 parts to represent 2⁄5. This leaves 3 parts unlabeled and 1⁄3 is simpler to identify.
Does the Read-Draw-Write process help us solve multI-step word problems Involving fractions? How?
Yes. We read, draw, and write in chunks.When we learn new information, we pause to draw and then go back to reading and draw when we learn something new. We can write expressions or equations as we realize which operations we can use to find unknown information.
Yes. The model I draw helps me decide which operation to use to find an unknown value. Each time we find new information by evaluating an expression, we can compare it to our model and ask, Does that make sense based on what I see in the model?
In Lesson 21, students encounter additional multi-step word problems with fractional amounts and take on more responsibility in determining how to represent and solve. When students use a self-selected method to solve a comparison word problem involving fractions, they model with mathematics and practice Standard for Mathematical Practice 4 (MP4). The key question of the lesson: Does the Read–Draw–Write process help us solve multi-step word problems? How? has them reflecting on the process throughout the lesson and finally in the Debrief, as shown. The flexibility of the process supports students as they read and return to modify their drawings multiple times. RDW also continues to aid students in identifying the known and unknown information and a solution path.
Representing comparisons and fractional amounts in Grade Levels 4 and 5 prepares students to visually represent ratios in Grade Levels 6 and beyond. The number choices and problem types continue to grow in complexity over the years. However, the RDW process remains a foundational tool for solving word problems.
Grade Levels 6–Algebra I
Much of the progression of mathematics in the middle school years includes algebraic understanding and representation, and the RDW routine progresses similarly. Instead of using drawing as the main problem-solving strategy, Eureka Math2 Story of Ratios uses algebraic thinking as the main strategy. RDW progresses to RRSS: Read, Represent, Solve, Summarize. The representation can still be a drawing, but it could also be an equation with variables representing the unknown.
The first instance of RRSS in A Story of Ratios is in Grade Level 6, Module 1, Lesson 21. Topic D introduces rates and comparing rates, including solving multi-step rate problems in Lesson 21. In this lesson, students watch a video of the moon landing in 1969 and then a horse racing to the moon where the RRSS routine is introduced.
The Read portion of the routine is used to find the same information as in RDW, to identify what the problem is asking us to find and what we know. In the Represent portion of the routine, students choose the representation that makes the most sense to them, such as tape diagrams, a double number line, a table, or a graph, and then they share their representations with one another. Pairs then use their representation to solve the problem. After completing the problem, the teacher directs students to summarize their findings by asking questions such as, “Does my answer make sense?” and “Does my result answer the question?”
Have groups work to solve problem 3. Circulate as students work, and observe the strategies groups use as they read, represent, solve, and summarize. Encourage students to return to problems 1 and 2 if necessary, and ask the following questions to advance their thinking:
- What is the problem asking you to find? What do you know that is given in the problem? What do you know based on your chosen method of travel?
- What tool can you use to represent the problem?
- Does your model show what is known and whot is unknown? How can you improve your model?
- What units do you need to consider in the problem?
- Does your answer make sense?
- Does your result answer the question?
To continue practicing the routine, students choose from a list of transportation methods and determine how long it will take for that method to transport them to the moon. The teacher is given a list of possible questions to advance students’ use of the RRSS routine. Beyond the Read portion of the routine, questions such as “What tool can you use to represent the problem?” and “Does your model show what is known and what is unknown?” gently guide students through the Represent portion of the routine.
This lesson that specifies the use of the RRSS routine is rare. Generally, the routine is not called out in the problem prompts or lesson structures. Instead, the tool is part of the students’ toolkit, and students reach for it when they deem it appropriate. Because of this, teachers will likely need to post the steps to the routine, say the steps during think-alouds, and remind students during problem-solving activities. Consider using the advancing questions from Lesson 21 in other problem-solving situations.
The first instance of the routine in Grade Level 7 is in Module 1, Lesson 11, when students are further encouraged to represent the problem “…by using a tape diagram, an equation, a graph, a table, or any other model.” Advancing students’ thinking toward an algebraic representation, students use a double number line, then a graph, and finally an equation for the distance formula, d=rt, in subsequent problems in the lesson. The RRSS routine is mentioned in Launch and in a Teacher Note but not called out further in the lesson guidance. Encouraging students to use RRSS as a tool in their kit will again be important for Grade Level 7 teachers.
There are many instances in Grade Level 8 when using the RRSS routine would aid students in problem-solving. However, the routine is not called out by name until Module 4, Lesson 10. In this Grade Level 8 lesson, students use linear equations to solve real-world problems. Students begin working with the routine as a class. Then, they use the routine with a partner and eventually work with it independently. While other representations may help students write the linear equation, representation of the problem with a linear equation is a specific task requirement. Students analyze the number of solutions to the equation in the context of the situation as they summarize the solution in the last step of the routine. Students recognize that the solution to the equation does not always answer the question from the problem, an additional complexity to problem-solving at this level.
The work with Read–Draw–Write and Read–Represent–Solve–Summarize engages students in many of the mathematical practices, but ultimately leads to the final goal, success with the modeling cycle (MP4) for problem-solving in high school mathematics. Math Practice 4 begins with, “Mathematically proficient students can apply the mathematics they know to solve problems arising in everyday life, society, and the workplace.”1 There are many ways to model the process of mathematical problem-solving. Most start with a real-world problem, iterate, formulating a solution strategy, performing some computations, interpreting the results, ensuring those results are accurate, and then reporting final results if they are validated.
The Algebra I Eureka Math2 curriculum takes the modeling cycle to its fruition, giving students multiple experiences with real-world problems. The first instance is in Module 1, Lesson 7 titled Printing Presses. Students work in groups to find an entry point for solving a problem about printing presses. Students analyze a variety of solution paths, building connections between quantitative reasoning and the process of writing and solving an equation in one variable. Connections are made to the RDW and RRSS routines when the teacher asks, “What assumptions can be made? What information do we know remains constant?” and “What are the important quantities? How are these quantities related?” The answers to all of these questions result in ways to read and represent the information from the problem.
In Module 2, students watch a video showing a “regular” showerhead and a low-flow showerhead. To further develop the modeling cycle, instead of giving students the problem, students explore questions related to the problem before narrowing their focus to one question. Information is withheld to allow students to independently determine what is required to answer the question. Additional information is provided only after students decide what assumptions need to be made during the formulation and computation stages of the modeling cycle. Students eventually connect different solution paths to a system of linear equations and explore the effects of changing the assumptions within the context as groups interpret and verify their results.
In other lessons, Algebra I students analyze falling objects and projectile motion, maximize area, and determine how a search and rescue helicopter relates quadratic functions to the real world. Connecting linear and exponential functions to the real world involves lessons in which students work with world populations, temperatures of objects cooling over time, and invasive species populations. The final Module of Algebra I brings all the years’ learning together as students use the modeling cycle to analyze paint splatters, reflect on the role of a city planner, consider a financial deal proposed by a business owner, plan a three-dimensional model of the solar system, and plan a fundraiser that maximizes profit.
Download the Article as a Free PDF
Great Minds
Great Minds PBC is a public benefit corporation and a subsidiary of Great Minds, a nonprofit organization. A group of education leaders founded Great Minds® in 2007 to advocate for a more content-rich, comprehensive education for all children. In pursuit of that mission, Great Minds brings together teachers and scholars to create exemplary instructional materials that provide joyful rigor to learning, spark and reward curiosity, and impart knowledge with equal parts delight.
Topics: Featured Math Concepts Conceptual Understanding Eureka Math Squared
Get the Latest Great Minds Updates
Stay informed of simple yet crucial implementation support tips, curriculum updates, new resources, professional development opportunities, and more for math, science, and ELA.
