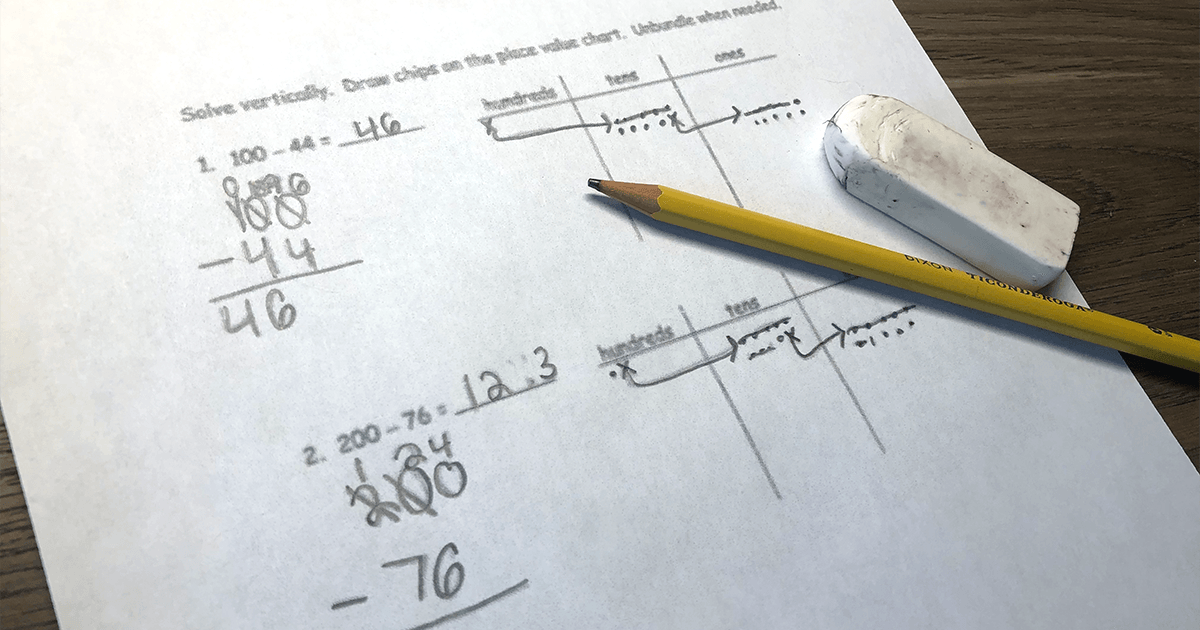Posted in: Aha! Blog > Eureka Math Blog > Implementation Support > Resisting the Urge to Reteach for Mastery
We all want our students to get it. Not the fifth time…the first time. We want to be wowed, to marvel in their successes, to witness that deep conceptual understanding called for by today’s mathematical standards. Often, when our students don’t demonstrate mastery, we feel deflated and discouraged. Sometimes we blame ourselves and sometimes we point fingers. But inevitably, we brush ourselves off, steadfast in our resolve to re-teach. Today, my students will get it.
What drives this urge to reteach? For me, it’s simple: discomfort and fear.
My kids didn’t get it yesterday, and I’m afraid they won’t if I move on. I know it’s important for the test. I don’t want my students to fail. I need to hammer it home.
The reality is that when most students are thinking hard and wrestling with new concepts, their work isn’t stellar. It often reveals misconceptions or gaps in understanding that cause our discomfort. When this occurs, how can we resist the temptation to reteach?
ANALYZE EXIT TICKETS
At the conclusion of every Debrief, students complete an Exit Ticket to demonstrate their understanding of the day’s lesson. Exit Tickets are meant to take a class temperature: How are my students doing? What are their strengths? Are there common pitfalls or misunderstandings? This doesn’t have to take more than a couple minutes. Look at the commonalities and create a few piles to distinguish levels of understanding
Let’s examine a Grade 2 Exit Ticket (Module 4-Lesson 27) together and develop a plan of action.

What does this student know?
- In both cases, she knows that she needs to decompose a hundred in order to subtract.
- Her chip models show that 1 hundred can be decomposed into 10 tens, and 1 ten can be decomposed into 10 ones.
- She knows how to subtract using the chip model.
Where is this student struggling?
- The unbundling (i.e., renaming) using the chip model does not match the vertical written method.
- The written method does not indicate that this student conceptually understands what is means to rename 100 or 200.
While this student hasn’t demonstrated a conceptual understanding where we can confidently check the “got it” column, she is on her way. There is a difference between finding success and demonstrating full mastery. And, I would argue that many of us came to the teaching profession to witness the beauty inherent in the journey of getting it. Learning takes time. This student is growing. Her Exit Ticket indicates that she does not need to be retaught the concept; rather, she needs more practice and experience with it.
CUSTOMIZE THE NEXT DAY’S FLUENCY
Not surprisingly, the misunderstandings demonstrated by this Grade 2 student were common among her classmates. In general, Exit Tickets showed that students didn’t yet fully understand what it means to rename with zeros and set up for subtraction. My solution was to customize the next day’s Fluency to address this shortcoming.
It’s no coincidence that one of the next day’s fluency activities is called “Rename the Units: Choral Response” (2.NBT.1). Below is a short snippet:

In addition to the choral response, I had students write this on personal white boards in preparation for the vertical form. I also omitted one of the other suggested activities that students already did well. In my opinion, the 10 minutes allotted for fluency practice is the best way to revisit previous concepts and gradually iron out the wrinkles without losing momentum.
It’s important to point out that because renaming with zeros is a challenging concept, Lesson 28 is intentionally designed for students to practice concepts taught in Lesson 27. Did two days iron out all the wrinkles? No. But, it did take care of some.
TRUST THE CYCLIC NATURE OF THE CURRICULUM
Here’s the good news: The Eureka Math curriculum was designed to continually revisit concepts. We circle back to previously introduced concepts in later modules. Famous cognitive theorist, Jerome Bruner (1960), believed in the power of revisiting the same topic or theme several times so that each time, information is reinforced and solidified. Deep conceptual understanding doesn’t happen overnight, or in one or two lessons. It takes time for ideas and concepts to marinate
The cyclic nature of the Eureka curriculum is evidenced in many ways. You will notice how Grade 2 Module 5 builds upon Module 4, with the added complexity of students now adding and subtracting with larger numbers. In Lessons 16–17, specifically, students will revisit subtracting from multiples of 100 and from numbers with zero in the tens place. This presents another opportunity for students to put newly acquired knowledge into practice. Moreover, notice that application problems throughout the curriculum provide ample opportunities for students to practice new skills, strategies, and concepts in context.
It’s important to note that Grade 2 students are expected to add and subtract using concrete models, drawings, and strategies; however, fluently adding and subtracting multi-digit numbers using the standard algorithm is not a requirement until Grade 4 (4.NBT.4). There is time. Right now in your classroom, there are minds hard at work, growing and developing. Don’t stop to reteach for mastery if your students are finding success. Analyze, customize, and trust the learning process.
Submit the Form to Print

MaryJo Wieland
Topics: Implementation Support