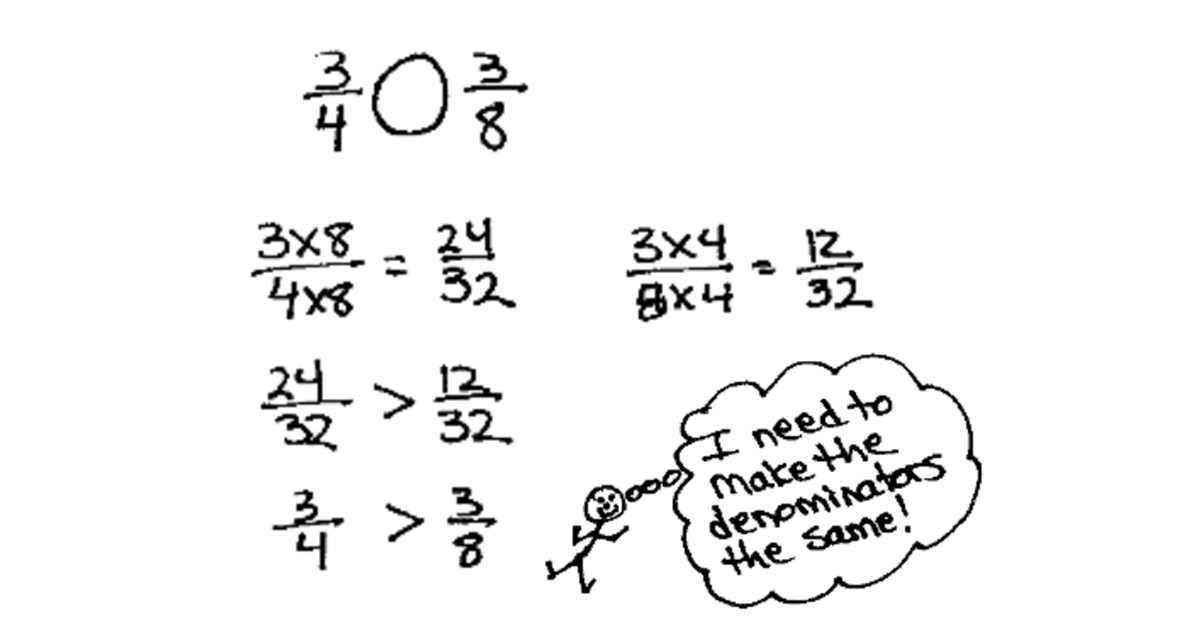Posted in: Aha! Blog > Eureka Math Blog > Conceptual Understanding > Comparison of Fractions — Beyond Common Denominators
STRATEGIES
Given two fractions, how do we compare them? As a young student, the way I learned to compare fractions was to use the strategy of finding common denominators. To complete the process, I simply multiplied the numerator and denominator of the first fraction by the denominator of the second fraction, and then repeated the process by multiplying the numerator and denominator of the second fraction by the denominator of the first fraction. Later, I was taught the “shortcut” of cross multiplication. Both strategies focused on procedural computation with little, if any, understanding of the process.

Fast forward to the Common Core State Standards. Does comparison of fractions look the same way it did years ago? Well sort of, but students are now presented with multiple strategies instead of just one or two, and not all of them are computational in nature. Now, comparison is about building understanding of fractions and then using that conceptual understanding to reason about the size of fractions.
Example 1:
Consider comparison of the following two fractions.
3/4 ___ 3/8

If I were to use the strategy that I was taught in elementary school, I would multiply the 3 and the 4, within 3/4, each by 8 to result in 24/32. Then I would multiply the 3 and the 8 within 3/8 each by 4 to result in 12/32.

Using cross multiplication is essentially the same process as above (in this case, making common units of thirty-seconds). The difference is that there is no mention of the unit; instead there is an emphasis on the quantity of the unit.

Now, think about the same comparison, noticing that the denominators are related denominators (i.e., 4 is a factor of 8). We rename 3/4 in terms of eighths. 3/4 is equivalent to 6/8. 6/8 is greater than 3/8, so 3/4 is greater than 3/8.
Is using one of the above strategies necessary to complete the comparison? As you have likely responded, the answer is no. Consider the following alternative strategies that illustrate a deeper understanding of fractions.

To show 3/4, a tape diagram can be drawn to model the fraction. Each fourth can be decomposed into two equal parts to show eighths. It’s easy then, visually, to see that 3/4 > 3/8 (note that this may not be the best strategy to use, however, when the denominators of the fractions being compared are not related).
Similar to the fraction model, a number line can be drawn to model the comparison. Fourths are decomposed to show eighths. 3/4 and 3/8 are labeled, and we can see that the distance from 0 to 3/4 is greater than the distance from 0 to 3/8.

Now consider focusing on common numerators, a strategy that is more abstract in nature. Notice that the units (fourths and eighths) are different, but the quantity of each unit is the same. Since the unit of fourths is greater in size than the unit of eighths, and since there are 3 of each unit, 3/4 is greater than 3/8.

The same comparison can be made using benchmarks fractions. To do this, first reason about the size of 3/4 and 3/8 in comparison to the benchmark of 1/2. 2/4 is equivalent to 1/2, so 3/4 is greater than 1/2. 4/8 is equivalent to 1/2, so 3/8 is less than 1/2. We can reason, therefore (by comparing each to the familiar 1/2), that 3/4 > 3/8.

Example 2:
Now, consider the following comparison.
2/3 ___ 6/8
Again, the focus of this comparison is common numerators; this time, however, the numerators are related (i.e., 2 is a factor of 6).

In 2/3, the unit is thirds and there are two of them. To make common numerators, we need 3 times as many as 2 (resulting in 6). If there are 3 times as many, each third will be decomposed into 3 equal parts, resulting in ninths. 2/3 is equivalent to 6/9. Since ninths are a smaller unit than eighths, 6/9 < 6/8. Therefore 2/3 < 6/8.

Example 3:
Ready for another example? How about 2/3 ____ 7/8? In this example, neither the numerators nor denominators are related. Consider the benchmark of 1 and the relationship of each fraction to 1. 2/3 is 1/3 from 1. Thirds are greater than eighths, so 1/3 is a greater distance from 1 than 1/8 is from 1. Therefore, 2/3 is less than 7/8.

The aforementioned strategies may be new to you. They were certainly new to me as an adult learner and opened my eyes to a new level of thinking. Consider taking some time to think about each strategy and how it lends itself to higher-level thinking and to a deeper understanding of fractions. Then, think about how each strategy can be used by students to help reason about the size of fractions before comparing them. If you would like to see these strategies in action, refer to Lessons 12–15 of Grade 4, Module 5.
This post is by Mary Swanson, a Grade 4 writer for the Eureka Math curriculum.
© Great Minds 2016
Submit the Form to Print

Mary Swanson
Topics: Conceptual Understanding