Student Voices: How Eureka Math® Has Shaped Two Students’ Math Education

Nell McAnelly, chair of the board of directors for the Great Minds® Public Benefit Corporation, sat down with two grade 9 students, Emma and Bella, to chat about their experiences learning math throughout their years in school. In elementary school, Emma and Bella learned with Eureka Math, which laid a strong foundation for their understanding of fundamental math concepts. As Bella and Emma progressed to middle and high school, they faced changing math curricula, different approaches to math instruction, and the COVID-19 pandemic—all of which have influenced the trajectory of their learning.

Read on for their observations, successes and challenges, and advice for educators. [Note: This interview has been edited for length and clarity.]

Were you taught mathematics the same way in elementary, middle, and high school?

Bella: No, it felt like through elementary school it was a lot more cohesive with a lot more strategies that were used to help us understand math more. With middle school it was really hard because it was online, and our teachers weren’t prepared to teach us through virtual learning, so it felt like they were trying to figure out new strategies to use and it didn’t work super well. And now in high school, we have several different teachers every year, and they all have different strategies and different ways to teach things. And of course, that’s going to happen, but the strategies don’t really carry over and it’s hard to pick up every single time you get a new teacher.

Nell: When you say your elementary school teachers taught you to understand math more, can you give me an example of how they might have taught you to understand math concepts?

Bella: I feel like the biggest thing I remember is multiplication—I know that for some people it’s just memorization, but for us it was understanding through groups of a number. Like I know that five times ten isn’t just fifty, it’s ten groups of five, and that’s easier for me to understand. And I have strategies like that for all the basic functions that help me understand them more now.

Nell: Emma, can you give an example of the way you were taught in elementary school?

Emma: I would say we learned a lot through repetitiveness. So learning something once and then using it in different situations. We did a lot of word problems to connect math to real life and just a lot of repetitive things that I think helped gain those skills and keep them in our brains throughout our education.

Nell: Did you use things like manipulatives or any kinds of written models?

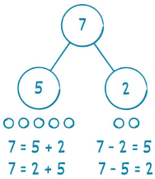
What is a Number Bond?
Number bonds are one of the most effective tools for modeling and recording composition and decomposition. They show the relationships between numbers, which is a key element of number sense, and provide a reliable visual structure that helps students learn basic facts more easily. Number bonds help students think flexibly about numbers and prepare students to do quick calculations in their head.

Bella: We used a lot of models, especially earlier on—things like tape diagrams and little graphs, and I feel like those definitely helped us understand everything we were doing, and it kind of stuck with us now.

Nell: When you manipulatives or tape diagrams or number bond diagrams, what happened after that?

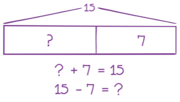
What is a Tape Diagram?
Like number bonds, tape diagrams can be used to show part–whole relationships, but tape diagrams are proportional and there is more variety in how they can be drawn and labeled. Tape diagrams are useful for solving many different types of problems but are commonly used with word problems. The use of tape diagrams supports students’ transition from concrete models to representational and symbolic models.

Bella: For me it feels like I’m not just picturing the number bonds in my head, I’m working through the number bonds in my head. Like when we were taught for addition that the number in the middle would separate off into two smaller circles, that’s what I picture in my head—they are coming together to be one number.

Emma: I would say the models that we used we still use now, especially the shapes and even the 3-D models when you add and subtract. I can think, “Okay, this is how much on this side, this is how much on that side,” and then, just like Bella said, visualizing what that would be.

Nell: Do your high school teachers use models?

Bella: Not in the same way. It’s mostly just visualizing the shape in geometry but not helping us work through and solve the problem in our head. I could still go back and use a lot of those diagrams that I used early on to help me, and I could maybe even come up with some that I could use to help me now, but they’re not really being taught to me anymore to help me understand what I’m doing.

What connections do you see within a grade and across grades as you’ve gone through elementary and then middle and now high school?

Bella: I felt like in elementary school math carried over a lot. It felt like, in a way, we picked up right where we left off each year. Now it feels like we are shifting focus every single year to a different topic, and then we have to think back to different grades to try to pull things in. And it’s harder to pull back that stuff we learned in middle school into our grade now because we skipped over shapes for a year, and now we’re back to them.

Nell: What do you think makes the difference in the connectedness that you’re expressing about elementary school and the difference in what you’re seeing now?

Emma: I think the difference in the connection is, in elementary school as Bella said, concepts connect from year to year. The teachers maybe talk to each other more, and we were all kind of on the same page. In high school now, different people are at different levels, and we’re not always in the same classroom to learn. And then, in the middle of my year in math this year, we changed curricula. It was really tough for me because we’d start one place and then we had to start over or go back to a different thing that we didn’t use before, so it didn’t really connect.

Bella: Yeah, I think throughout most of elementary school we had Eureka Math, and it was pretty stable throughout those five years. But we’ve had like four different math curricula since then, and it feels like not all of them are working together, or they kind of overlap, or you miss something. It’s a lot harder now, and I think that definitely has to do with the math curricula because we haven’t had the same math curriculum for more than a few years since then.

When you get to a roadblock or a stumbling point in mathematics, what helps you work your way through that?

Bella: Breaking down the problem. A lot of these harder problems that we’re doing now, underneath it is just all the math that we learned when we were little. So breaking it down and going from there helps us at least get part of the equation done. Then we can think back to what we just learned, about all the new things that are being added in, and think about that in the same way.

Making Sense of Complex Problems

“A lot of these harder problems that we’re doing now, underneath it is just all the math that we learned when we were little. So breaking it down and going from there helps us at least get part of the equation done. Then we can think back to what we just learned, about all the new things that are being added in, and think about that in the same way.”

—Bella, grade 9 student

Emma: Like Bella said, the harder problems are usually multi-step, so just breaking it down and trying it multiple times if you don’t succeed, and then maybe asking for help or checking it with a peer or something like that really helps you get past that roadblock.

Nell: How does your approach to handling roadblocks compare with the other students in your class that maybe didn’t have the same type of understanding of mathematics?

Bella: I think a lot of those kids who didn’t have that same basic understanding from when they were younger don’t know what to do to get past those problems. I could tell them to try and break it down, but even with that broken down version, they don’t have a solid understanding of it. So usually they’ll either ask for help to have someone walk them through it, or they’ll just give up because they don’t know what to do with it.

Are there other subjects in school that you see math applying to?

Bella: Definitely science. It felt like when we were younger, math and science were two completely different subjects, but now they blur together a little bit. Now on our science tests there are math questions that aren’t the math level we are learning now, but it’s math that we learned in later elementary school and early middle school that they’re calling back to in some of these questions. I think it was a good thing we learned it then and can understand and remember it now so that it makes science a lot easier for us.

Nell: How does your work in math and science compare to your fellow students that maybe had a different type of learning?

Bella: I think the students that didn’t necessarily learn those topics in depth when they were younger are having a harder time now, not just in math but in science, because for the next test one of the things they have to review is the math that’s on the test. So it makes it a lot easier for us just having that baseline of math from earlier.

Emma: Yeah, I agree. I think if we hadn’t had that baseline and in-depth learning of math, it wouldn’t come as easy to us as it does now, especially in science where we probably spend a lot more time reviewing and going over the math problems instead of focusing on the other science problems that we need to do.

If you had to describe math in just a few words or tell me something key about mathematics, what would you say?

Bella: I would say that math isn’t just math. It gives us more of a logical brain, an analytical brain, so that we can analyze things and make sense of our problems and work them through step by step.

Emma: I would say math is very much needed in anyone’s life. It has helped me with different things, like calculations and figuring out problems, and it has helped me gain confidence in school because once I figured it out, I could be like, “Oh, I can help this peer or that peer.”

Nell: That’s great, knowing that confidence and logic are other side effects of a good mathematics program—it’s really building a life skill.

If you could give advice to younger students, or if you could give feedback to your teachers or the administrators, what would you like for them to know?

Bella: I wish that we had stayed in the same curriculum from elementary school to middle school. I wish our teachers and administrators had worked together more from school to school because when we went from our last year of elementary school to our first year of middle school, it felt like it was completely different because we hadn’t had a new curriculum before. Then we were getting this new curriculum, and it just didn’t seem to work. So I wish that our administrators had worked together to make that transition better.

Emma: I would say for students: Just keep working at it, and don’t give up on it. If it’s hard, ask for help—don’t be ashamed asking for help. Just keep trying at it because soon you’ll get it.