Posted in: Aha! Blog > Eureka Math Blog > Instructional Design > The Progression Of Grade 4 Modules
FAQ RESPONSE
Consider the following as an example of the intentional trajectory of the modules within A Story of Units. Here, Grade 4 is used to demonstrate coherence and to show how students gain a deeper understanding of mathematics
MODULE 1:
Grade 4 Module 1 builds number sense and also gives students access to the algorithms for addition and subtraction that allows them to reach the grade-level fluency standard of addition and subtraction by the end of the year. Students extend their prior work with place value, rounding, and solution strategies for addition and subtraction as they work with larger numbers. Work with the familiar units of ones, tens, hundreds, and thousands is extended to ten thousands, hundred thousands, and 1 million. Additionally, the continued use of unit language, foundational from prior grades, is stressed and benefits student understanding in future modules.
MODULE 2:
Although a short module, Module 2 was carefully and purposefully created and placed with direct relation to Module 1. In this module, students use the standard algorithms for addition and subtraction and see how use of the algorithms has direct application to units of measure (e.g., centimeters, meters, and kilometers; grams and kilograms; and liters and milliliters). Students manipulate units and use place value understanding to convert from larger to smaller units (e.g., 1 meter = 100 centimeters), and they see the relationship of the units of thousands and ones to the units of kilometers and meters, kilograms and grams, and liters and milliliters. Module 2’s work is foundational and directly related to Modules 3, 5, and 6. In Module 3, for example, prior work with conversions helps students to discover patterns of “ten times as many.” In Module 5, students work with fractional unit relationships (e.g. 1 fourth = 2 eighths), and in Module 6, place value relationships further develop and extend into tenths and hundredths, finding 1 one to be 10 times as much as 1 tenth.
Given that Module 2 is such a short module and that the measurement standards within Grade 4 are considered (as outlined in this resource from Student Achievement Partners) to be ‘supporting’ and ‘additional’ ‘supporting’ and ‘additional’, some teachers, because of time constraints, have chosen to skip Module 2. However, in speaking with teachers who have done so, we often heard that mid-way through Module 3 they had to backtrack to further solidify place value understanding. Those teachers who had taught Module 2 found that the backtracking was not necessary. In other words, skipping Module 2 (in the end) didn’t save any time
MODULE 3:
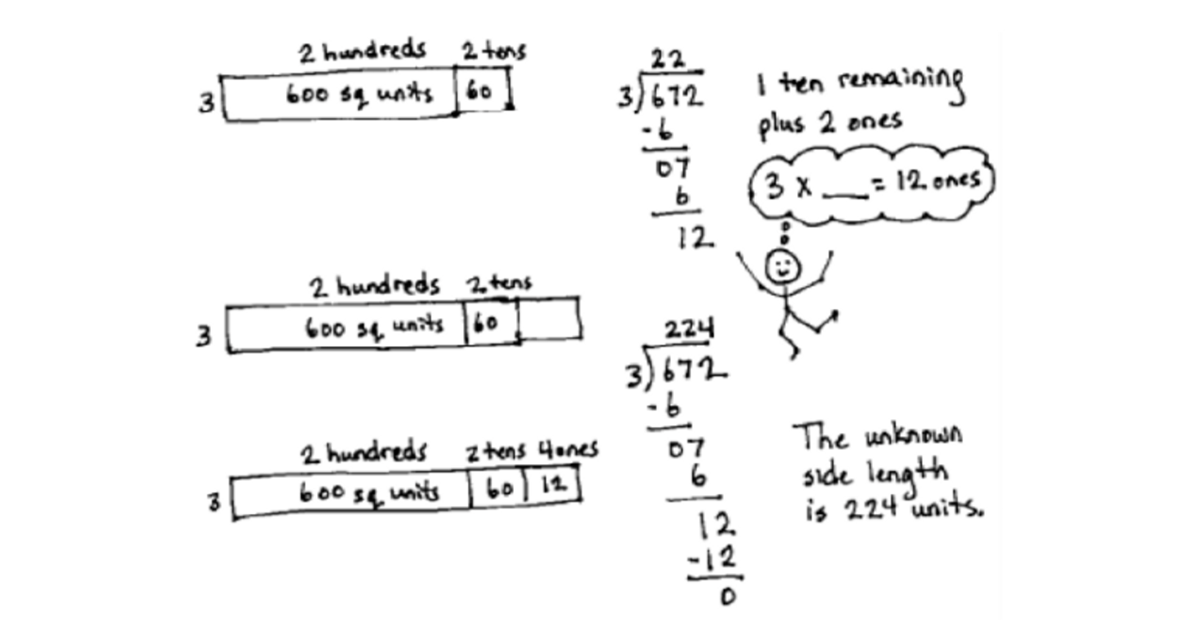
Module 3’s work with multiplication and division comes next. Again, this module was strategically placed. Students begin with lessons on area and perimeter; this relates back to their use of units of measure within Module 2 and is one way that multiplication and division culminate in Grade 3. Throughout Module 3, familiar models such as arrays, the area model, and tape diagrams are used during the problem-solving process. Units are manipulated as students work toward success with multiplying two-digit by two-digit numbers and dividing a multi-digit number by a single-digit number.

For example, the unit language of ones × ones, ones × tens, ones × hundreds, tens × tens, and thousands, hundreds, tens, and ones divided by ones is stressed in order to build conceptual understanding of the multiplication and division process. Additionally, students use place value understanding to show regroupings within the written methods (e.g., 18 tens is recorded as 1 hundred 8 tens).


MODULE 4:
Module 4’s placement aligns with the pre- and post-test standards of New York State and also allows students a mental break after the intense work with multiplication and division in Module 3. Students identify and draw points, segments, lines, rays, acute angles, right angles, obtuse angles, lines of symmetry, parallel lines, and perpendicular lines. They use a protractor to measure angles and develop an understanding of the unit of a degree. Students compose and decompose angles, thus strengthening their addition and subtraction skills and their reasoning skills. Finally, students define and construct triangles and quadrilaterals based on given attributes. Given the unique nature of Module 4, you may wish to consult the Pacing and Preparation Guide, which explains the flexibility of where Module 4 can be taught given that the work completed in Module 4 is foundational to geometry concepts of higher grade levels
MODULES 5 & 6:
In Module 5, students learn fraction equivalence, ordering, and operations. Armed with an understanding of units, students naturally see fractions as numbers on the number line and are able to make sense of equivalency of fractions and operations with fractions. Just as whole numbers exist on the number line, so do fractions. This learning naturally extends to Module 6 where students learn that decimal numbers are simply another way to express fractions having units of tenths and hundredths. Students complete operations with decimal numbers by renaming them as fractions, completing the operation, and then converting back to decimal form.

MODULE 7:
Module 7, the culminating module of Grade 4, builds on the work of Module 2 as students once again work with the conversion of units. Students work with US measurement units related to time, length, weight, and capacity. Students convert various units such as pounds to ounces and yards to feet. Students use their multiplication skills from Module 3 as they complete these conversions that do not follow the same base-10 patterns as metric units. (Note that students without experience with Module 2 would be at a disadvantage in not having had the previous work with conversions.)
OTHER CONSIDERATIONS:
As you continue planning for your year and consider what is written here, also keep in mind that skipping or altering the order of the modules can snowball into other areas and bring about complexities and a need for further planning. The fluencies, for example, build from one module to the next. Alteration of the order of the modules would mean a disconnect in the purposefulness of the fluencies within the lessons. Challenge yourself to trust the intentionality of the modules and their coherence. It will all come together in the end.
This post is by Mary Swanson, a former teacher, who is a writer for the Eureka Math A Story of Units curriculum.
Submit the Form to Print

Mary Swanson
Topics: Instructional Design