Posted in: Aha! Blog > Eureka Math Blog > Conceptual Understanding > Rigid Motions From Grade 8 To 10
AHA! MOMENT
My three-year-old niece was practicing her flashcards the other night (one of her favorite night-time activities!). She grouped all the rectangles together, arranging them so they were all horizontal. I rotated one card and asked her if the shape was still a rectangle, she replied with an emphatic, “NO! It’s THIS way,” and changed the orientation so it looked correct in her eyes. My niece has a long way to go before understanding the meaning of similarity and congruence, but as children explore geometry through “A Story of Units,” they develop a more sophisticated understanding of this concept of basic rigid motions.
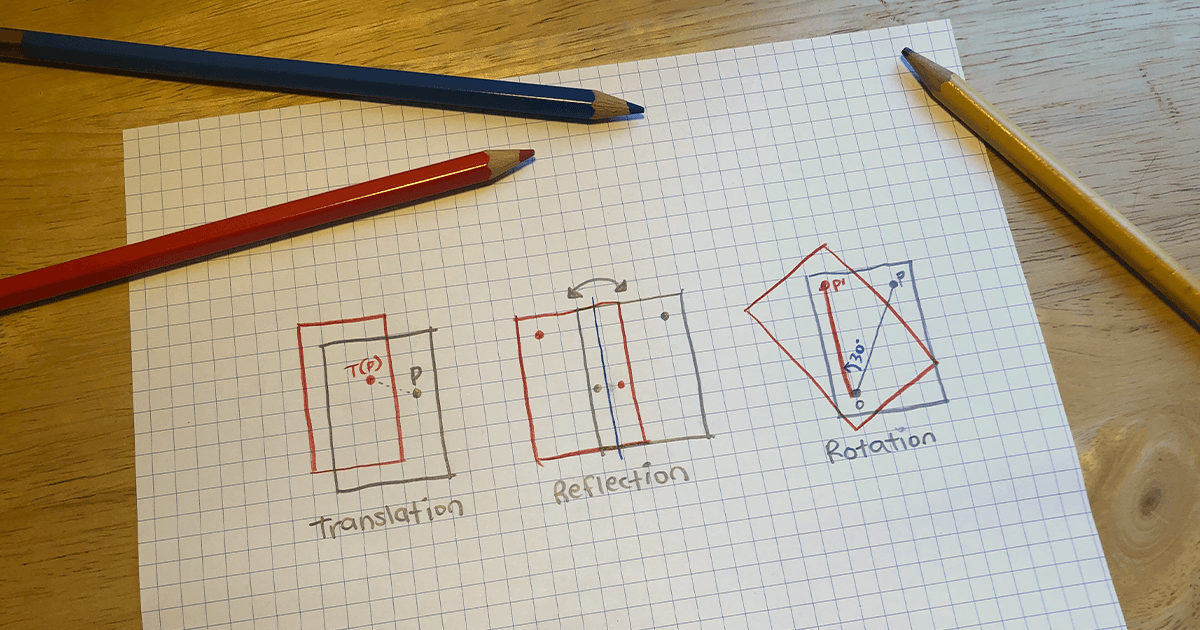
One of the major changes in geometry curriculum under the Common Core State Standards is the way congruence is defined using basic rigid motions. An example of coherence in the Eureka Math curriculum is the study of rigid motions in Grades 8 and 10. Students experience a transition from a pictorially based introduction to an abstract understanding.
The fundamentals of rigid motions are first introduced in Grade 8, Module 2. Students study translations, reflections, and rotations to build an intuitive understanding of each transformation. They are able to use a hands-on model of a rigid motion in order to describe the way each rigid motion behaves and list observable properties.
What does this transition look like? For example, in Grade 8, a reflection is modeled by laying a sheet of transparency over a sheet of paper of the same size with the following figures drawn on it: a line, a ray, a segment, an angle, and two labeled points; one point is on the line, and one point is not on the line. The sheet of transparency is laid over the paper, and all the figures are traced by marker. To model the reflection, students flip the transparency over, and re-align the line on the transparency with the line on the paper. They observe that the reflection has not changed the fact that a line is still a line, and similarly, the ray, the segment, and the angle are still each of those figures, respectively, in the reflected version. They measure the length of the reflected segment and the angle measure and see that the measures are unchanged.

Then in Grade 10, Module 1, precise language and notation is used to define each of the rigid motions. A reflection is formally defined:

Constructions studied in this module inform students why reflections behave the way they do, and the properties formerly observed are proven using deductive reasoning. Once the basic rigid motions are established, students are able to reason whether or not figures in the plane are congruent. The transition then, is a progression from hands-on model and observation to purposeful drawing and, finally, to careful and precise use of language.
Join the conversation about rotations, reflections, and translations on the official Eureka Math Geometry Facebook page.
The background image is from Grade 10, Module 1 in the Eureka Math Free PDF page, available at www.eureka-math.org.
This post is by Pia Mohsen, a former teacher, who is a Grade 10 writer for the Eureka Math curriculum.
Submit the Form to Print

Pia Mohsen
Pia graduated from the University of Pennsylvania with a Bachelor of Arts in Economics and a minor in Psychology. She taught high school math at the New York Harbor School for seven years through the New York City Teaching Fellows program. After moving to Philadelphia, she joined the Eureka Math team and helped write the first edition of Geometry for the curriculum. Outside of work, Pia enjoys getting crafty by making cards and finding new corners of Philadelphia to explore with her husband and two boys.
Topics: Conceptual Understanding