Posted in: Aha! Blog > Eureka Math Blog > Models > Application of the Tape Diagram—A Tool For Problem Solving
STRATEGIES
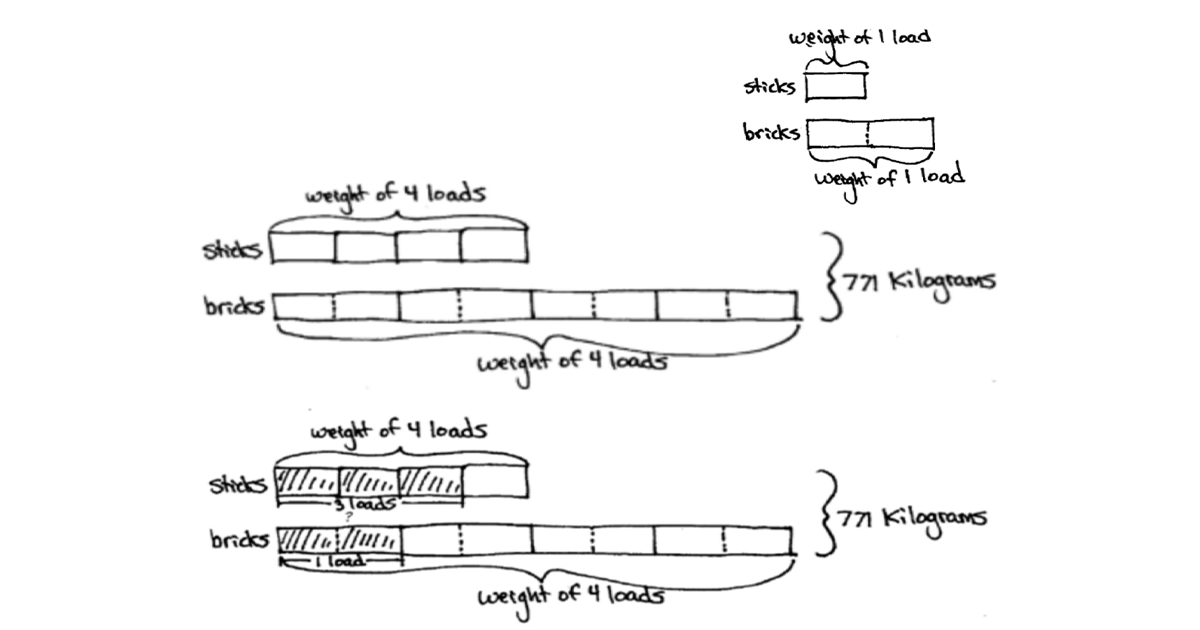
A load of bricks is twice as heavy as a load of sticks. The total weight of 4 loads of bricks and 4 loads of sticks is 771 kilograms. What is the total weight of 1 load of bricks and 3 loads of sticks? (Grade 5, Module 2, Lesson 28)
To solve this Grade 5 problem, my 9th grade daughter first divided 771 by 12 and then multiplied the quotient by 3 and by 2. She then added the two products together to get her answer. When asked why she did it that way, she explained (in one very long run-on sentence) that “since the bricks were twice the sticks and since there are 4 loads of each, that’s really 12, so I divided by 12, and then I multiplied by 3 and then by 2 (since the bricks are twice the sticks) and I added my answers together”. “But,” she concluded, “I have no idea how a 5th grader would do that!”
So, how would a 5th grader solve the problem? One way would be to follow the RDW process and to draw a tape diagram. Through the RDW process, the student would read and reread the problem, draw a tape diagram to help make sense of the information in the problem, solve the problem mathematically, write an answer statement, and then revisit the original problem to determine if his/her answer makes sense.
Consider the following dialogue as a possible interaction with a 5th grade student who is working to solve the problem. This example shows not only the direct power of the tape diagram, but it also shows the prior learning evident in the modules that brings students to the point where they can solve this problem with success.
A load of bricks is twice as heavy as a load of sticks. The total weight of 4 loads of bricks and 4 loads of sticks is 771 kilograms. What is the total weight of 1 load of bricks and 3 loads of sticks?
T: Reread the first sentence. What do we know?
S: We know that there are bricks and that there are sticks.
T: What do we know about the bricks and the sticks?
S: The bricks are heavier.
T: How much heavier?
S: Twice as heavy.
T: Let’s show that relationship using a tape diagram. Draw a tape to represent the weight of the sticks. Now draw another tape to represent the weight of the bricks. Which tape will be longer?
S: The tape for the bricks.
T: How much longer?
S: Twice as long.
T: Show me. Don’t forget to label.

T: What else do we know? Read on.
S: The total weight of 4 loads of bricks and 4 loads of sticks is 771 kilograms.
T: Can we draw something to represent that?
S: Yes. We can draw a tape diagram.
T: If this (pointing to original tape) represents the weight of 1 load of bricks, what would we draw to represent the weight of 4 loads of bricks? Show me.
S: We need to draw three more of that same size to show 4 loads.

T: How would we represent the weight of 4 loads of sticks?
S: We draw 3 more units.
T: Can we label anything else?
S: Yes. We can label the total. It’s 771 kilograms.
T: What do we need to find out?
S: The weight of 1 load of bricks and 3 loads of sticks.

T: Where do you see that in the tapes?
S: Here is 1 load of bricks. Here are 3 loads of sticks.
T: Let’s look back at the problem one more time. Have we drawn everything that we can draw?
S: Yes!
At this point, students have drawn a tape diagram to represent the problem, and they are ready to make a plan to solve.
T: The tape diagram helps us to figure out how to solve. What do you see?
S: I see 12 equal-sized units.
T: And what do we know about the units?
S: The total is 771 kilograms.
T: And what do we need to find out?
S: How much 5 of the units would be.
T: How do you know?
S: That’s how much 1 load of bricks and 3 loads of sticks is.
T: How can we determine how much 5 units is?
S: First, we need to figure out 1 unit. Then we can figure out how much 5 units would be. I’ll divide 771 by 12 to find 1 unit. Then I will multiply by 5 to find 5 units.
Students can now use their knowledge of division, multiplication, and addition to determine the answer.



S: My answer is 321.25. 1 load of bricks and 3 loads of sticks weigh 321.25 kilograms.
T: Does your answer make sense?
S: Yes, because the weight of 1 load of bricks and 3 loads of sticks is a little less than half the total weight. 321.25 kilograms is a reasonable answer.
Note that the essential reasoning by both the 9th grader and 5th grader are the same. Both draw the conclusion that 4 loads of bricks and 4 loads of sticks is equivalent to 12 loads of sticks and pursue the answer from there. The tape diagram serves as an access point for the 5th grader, whereas the 9th grader’s fluency with this reasoning allows for instantaneous articulation of the same concept.
Students are first introduced to the tape diagram in Lesson 19 of Module 4, Grade 1. Prior to that module, students are provided with the foundational skills that lead to an understanding of the part/whole relationship and what the tape diagram represents. The transition to the tape diagram is beautiful! If you’d like to see it in action, check out Topic E of Grade 1 — Module 4.
This post is by Eureka Math teacher-writer Mary Swanson.
© Great Minds 2016
Submit the Form to Print

Mary Swanson
Topics: Models