Posted in: Aha! Blog > Eureka Math Blog > Instructional Design > Eureka Math Lesson Structures
We are often asked for clarification about the differences between the elementary lesson structure and the secondary lesson structures. When preparing for instruction, it is certainly helpful to understand the differences in order to capitalize on the design.
A Story of Units (Grades PK-5)
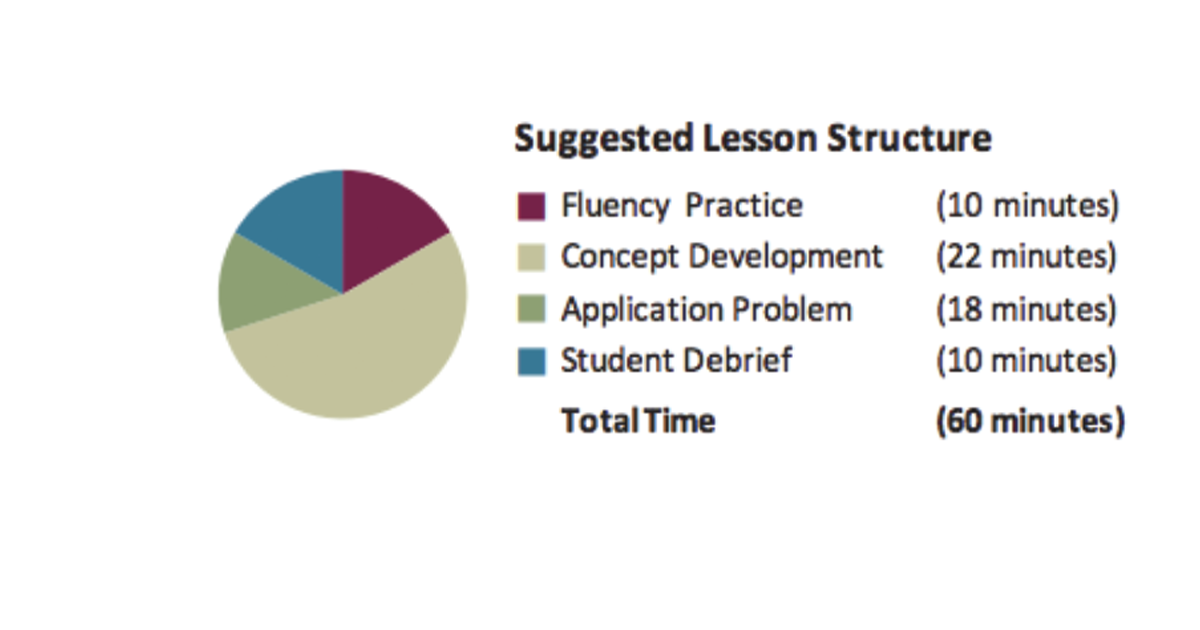
Each lesson in A Story of Units is comprised of four critical components: fluency practice, concept development (including the problem set), application problem, and student debrief (including the Exit Ticket). Each component described below serves a distinct purpose. Together they promote balanced and rigorous instruction.

Fluency Practice: Almost all lessons begin with this component to support development of fluency skills for maintenance (staying sharp on previously learned skills), preparation (targeted practice for the current lesson), and/or anticipation (skills that ensure that students will be ready for the in‐depth work of upcoming lessons). This component provides daily opportunities for students to gain confidence and motivation for continued learning.
Concept Development: This component addresses the new content being studied. Therefore, it is often allotted the majority of the instructional period to give students time for discussion and reflection. The concept development is generally comprised of carefully sequenced problems centered within a specific topic to begin developing mastery via gradual increases in complexity. It is also accompanied by an additional set of carefully crafted problems called the “problem set.” Teachers are encouraged to make choices within this set of problems to provide their students with generally about 10 minutes of additional practice.
Application Problem: In most lessons, this component is included to provide students with an opportunity to apply their skills and understandings in new ways. Sometimes the application precedes the concept development, functioning as a springboard into the new learning of the day. Often the application follows the concept development as an extension of learning.
Student Debrief: Every lesson closes with this critical component in which the teacher engages students in a whole-group discussion, challenging them to share their thinking and draw conclusions. This allows the teacher to gauge student understanding of the concept of the lesson, offering another chance for students to gain understanding before attempting the exit ticket.
A Story of Ratios (Grades 6–8) and A Story of Functions (Grades 9–12)
Mathematical content naturally increases in its complexity with each grade level. In order to address the level of difficulty at the secondary level, the formats of the lessons take on different forms starting in Grade 6. Each lesson is formatted as one of four types, each driven by the specific content of the lesson, including: problem set lessons, Socratic lessons, exploration lessons, and modeling lessons. (Notice that the term “problem set” arises as an entire lesson format in grades 6–12, leading to the need for clarification.)

Problem Set Lesson: This lesson format is the closest comparison to the lessons within A Story of Units. This format consists of teacher-led examples that are generally followed by guided exercises in which students apply their understanding to related problems. There are often short discussions within these lessons helping students make critical connections to develop understanding of concepts.
Socratic Lesson: Some content within the grades is of greater difficulty and it is necessary to maintain a dialogue with students to develop their understanding of such concepts. The Socratic lessons are primarily student/teacher discussions centered on the difficult concepts.
Exploration Lesson: Students are presented exploratory challenge(s) in the form of activities and/or exercises in which partners or small groups work toward achieving a common goal. Exploratory challenges comprise the majority of the lesson.
Modeling Lessons: A practice that intensifies with each grade in middle and high school mathematics is the ability to model mathematically. Many misinterpret modeling as the use of manipulatives to show how the mathematics works. However mathematical modelling actually refers to the use of mathematical models to solve problems that arise in the real world. The modeling lessons consist of well- or ill-defined application problems for students to complete. These problems involve the real world application of the mathematics that is learned in the classroom. The lessons are primarily reserved for high school, but there are at least three modeling tasks throughout each middle school grade level curriculum.
Through the course of this description, we’ve used the term “problem set” in more than one way. At grades 6–12 there is, as described previously, the problem set lesson. However, regardless of format, each K-12 lesson has an associated problem set, a supplemental set of problems based on the content of the current lesson. The major difference in the meaning of the term problem set lies in how the problem set is used. The PK-5 problem set is expected to be used as part of the classwork whereas the 6–12 problem set is generally expected to be used as independent practice at home. Either way, teachers are encouraged to be flexible in their use of items from the problem set to best meet the specific needs of their students to support their conceptual understanding.
Submit the Form to Print

Catriona Anderson
Topics: Instructional Design