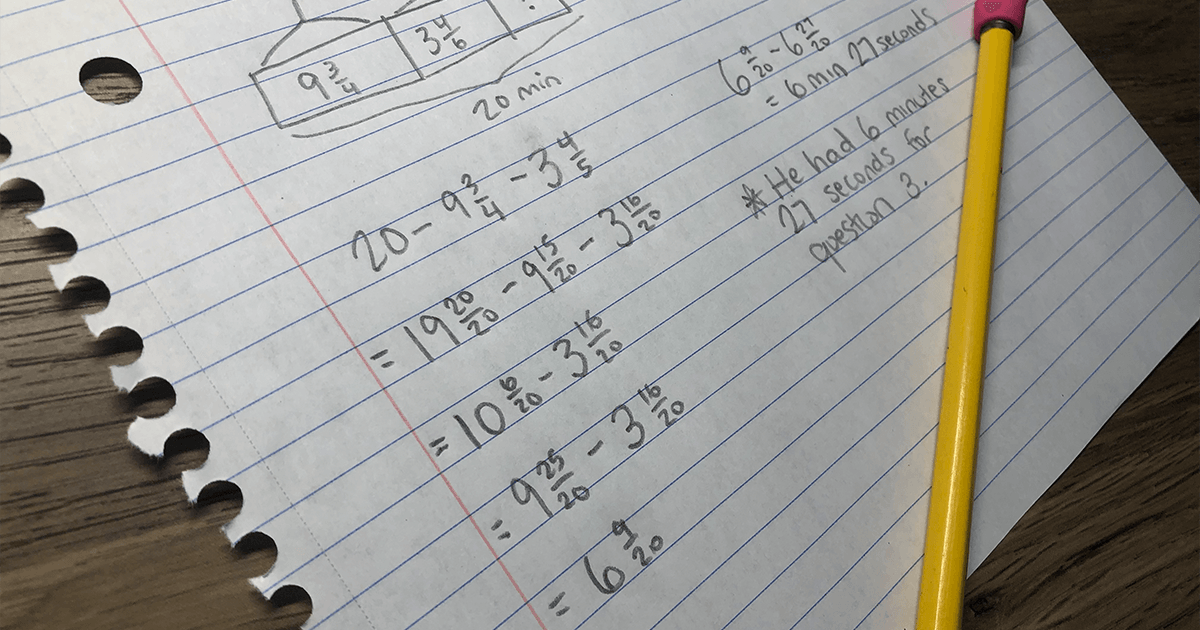Analyzing Student Work

STRATEGY

Although analyzing student work isn’t always easy, the benefits are invaluable. By studying our students’ approach to solving problems, we can discover new ways to teach a familiar topic, learn more about a particular child’s strengths and weaknesses, and plan modifications or adjustments in our current instruction.

Let’s walk through a student’s solution to Problem 4 of the Problem Set of Grade 5, Module 3, Lesson 15:

Problem 4

Gavin had 20 minutes to do a three-problem quiz. He spent 9 ¾ minutes on question 1 and 3 ⅘ minutes on question 2. How much time did he have left for question 3? Write the answer in minutes and seconds.

Step 1: The student drew a tape diagram. She knew to label the whole as 20 minutes, parts 1 and 2 with the number of minutes Gavin spent on those questions, and part 3 with a “?” to represent the unknown.

Step 2: The tape diagram helped her decide to subtract the amount of time Gavin spent on questions 1 and 2 from the total time spent on the quiz.

Step 3: Common units were formed and 20 was renamed. The student recognized the need for common units in this subtraction problem and was able to correctly determine the equivalent fractions. Why was the value of 20 renamed? She recognized that it would be too challenging to subtract the mixed fractions from 20, so 20 was decomposed into 19 and 1. The number 1 was renamed 20/20. Now, she is able to subtract.

Step 4: The student subtracted the first two mixed numbers in step 3. To do so, she first subtracted whole numbers, 19 and 9, to get a value of 10 and then subtracted the fractions to find a difference. Finally, she added 10 and 5/20.

Step 5: Because there weren’t enough twentieths in 5/20 to subtract 16/20, she unbundled a 1 to make 9 and 20/20, which became 9 25/20 when she added 5/20.

Step 6: After subtracting the two mixed numbers in step 5 the student remembered the answer needed to be written in minutes and seconds. She knew there are 60 seconds in a minute. Renaming the fraction as sixtieths made it easier to determine the final answer, which she wrote as a statement: Gavin had 6 minutes 27 seconds for question 3.

In analyzing this work and breaking down what this student was thinking in each part of the solution, we learned more about the knowledge and skills needed to successfully answer this problem. This student needed to know how to apply the “Read, Draw, Write” process, rename units, subtract mixed numbers, rename a fraction greater than one, and decompose or unbundle units in a way that simplifies problem-solving. The analysis here helps us anticipate where a student might get held up and allows us to customize our lessons to fit the needs of our students.

Looking at these steps also reveals alternative ways to solve the same problem, such as the following:

Although many of the steps in this alternate approach are the same as the steps detailed above, the strategy is different. Encouraging and accepting multiple strategies is a wonderful practice to incorporate into your math classroom because it addresses different learning styles. It also allows students to create their own strategy, which increases motivation to solve problems and strengthens their mathematical thinking when asked to explain or justify their work. Celebrating multiple solutions in our classrooms also creates a sense of excitement and fosters positive feelings toward math.

Planning the time to analyze student work has major impacts on our instruction and the success of our students. This can also be a great exercise to do in collaboration with colleagues. You may gain a different perspective, discover new ideas, and even increase your own understanding of mathematics.

This post is by Dawn Pensack who is a Grades 5–6 Eureka Math writer. She taught math for 10 years (mostly in Grade 6).