Teaching Addition With Integers Through the Integer Game

SPOTLIGHT A RESOURCE

In the past, eighth-grade teachers always asked if the seventh graders could spend more time working on operations with rational numbers because this was often a weakness for their students. As a seventh grade teacher, I quickly became frustrated to hear this same message year after year and I began to dread teaching students about operations with rational numbers. Every year I would hear the same questions: “Why are we subtracting when it says to add?,” “Why is the product positive when both factors are negative?” and “Is this a subtraction sign or a negative sign?” It did not take long for me to realize that asking students to memorize a bunch of rules was not a successful way to teach operations with rational numbers. To fully understand this concept, students needed some context. The Integer Game provides that needed context and gives students a tool to help answer these questions.

Below you will find an image of the description of the game, the rules and the cards. These are located in the Teacher Materials for Grade 7 Module 2

At first, the Integer Game is used to combine integers, helping students justify that an integer plus its opposite add to zero. Using the Integer Game to review opposite values is a great way to get students familiar with the game without struggling with the math concepts. Below are two problems from the Exit Ticket that enable teachers to assess students understanding of this idea.

Grade 7 Module 2 Lesson 1 Exit Ticket #’s 1 and 3

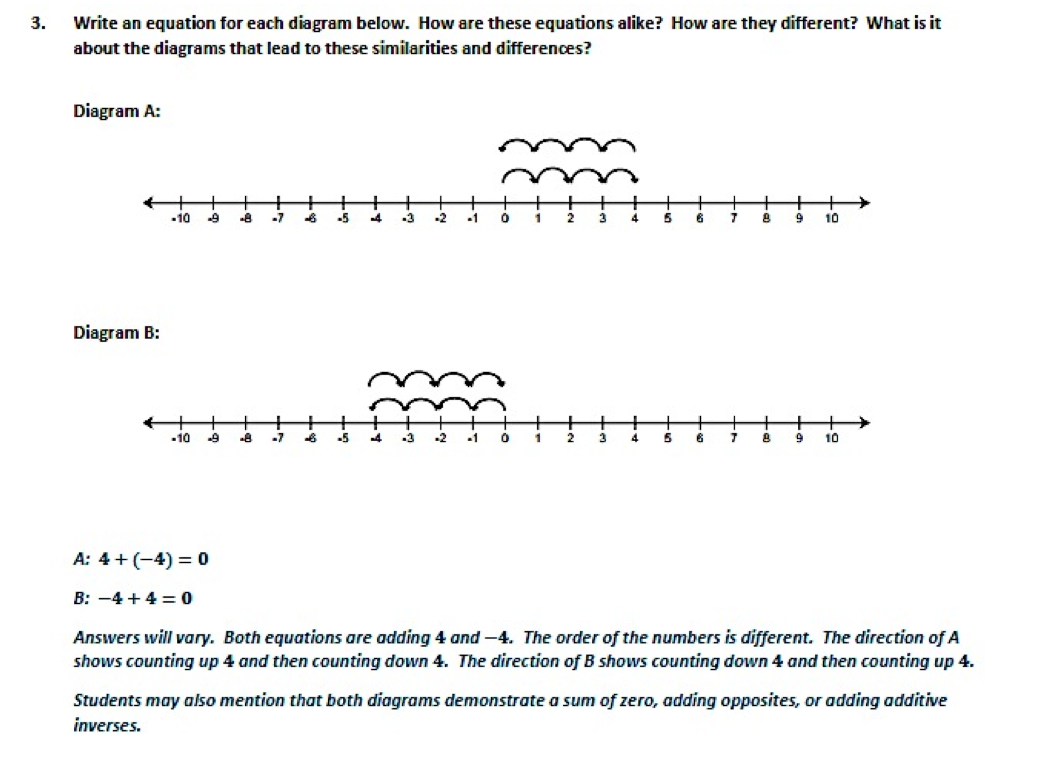
In Lesson 2, students use the number line to model the addition of integers. Notice how students are guided step-by-step through the process in Example 1 shown below. Also, notice the use of the vector, which is new to students.

Using vectors and a number line add increasing layers of complexity to the Integer Game when students start calculating the sum of their cards in Lesson 3: Understanding Addition of Integers. Even though students enjoyed the game, I was usually nervous about whether or not students could add integers, using their knowledge from their experiences with the game, but without the physical game.

Since I previously taught operations with integers through rules and the retention was often poor, I anxiously waited to see if my students could transfer their knowledge of integers through their experiences with the game to being able to accurately and efficiently calculate the sums of integers and other rational numbers. I was pleasantly surprised during the next few lessons, when students were able to calculate the sums of larger integers and different rational numbers. This proved to me that they had developed a true understanding of the process, which often led them to discover patterns of their own and, ultimately, the rules. Now, I was actually looking forward to teaching students about other operations with integers. Finally, my students weren’t memorizing rules because I said they were important. Instead, students had a context to explain how to evaluate expressions with integers and rational numbers.

Now, I have confidence with my students’ ability to successfully compete operations with rational numbers and I can’t wait to hear about it from the eighth grade teachers.

This blog post is authored by Krysta Gibbs, a Eureka Math middle school teacher-writer